Notes on Solid State (Integrated Standard) 

          

    A solid is defined as that form of matter which possesses rigidity and accordingly definite shape and definite volume.

    The state of a matter depends on two opposing factors namely the intermolecular force of attraction and the thermal energy (the energy possessed by the constituent particles due to temperature). 

    Gaseous substances have weak intermolecular forces and high thermal energy. But solid substances have strong intermolecular forces and low thermal energy.

General characteristics of solids:

1. Possess rigidity

2.  Have definite shape and definite volume

3. Have high density

4. Are incompressible.

5. Particles are fixed at their position and only oscillate about their mean position.

Classification of solids on the basis of arrangement of constituent particles:

Crystalline Substance: 

    

    A substance is said to be crystalline if its constituent particles (atoms/molecules/ions) are arranged in a definite geometric pattern in three dimensional space to have a long range order. For this reason crystalline solids have definite geometrical shapes.

Amorphous Substance: 

    A substance in which constituent particles are arranged in such a way that the regularity of arrangement is of short range order is called a amorphous substance. For this reason amorphous solids have no definite geometrical shapes.

Anisotropic effect in Crystalline substances:

    Though crystalline substance has regular arrangement of particles, the arrangement is not the same in all directions. Thus the properties like electrical conductivity, refractive index, thermal expansion, etc. have different values in different directions of the substance. This property of the material is called anisotropy and crystalline substance are said to be anisotropic by nature.

    

    On the other hand, particles in amorphous substances are not regularly arranged hence the values of properties like electrical conductivity, refractive index, thermal expansion, etc. are identical in all directions of the substance. Thus amorphous substances are isotropic by nature.

Difference between Crystalline and Amorphous substance: 

Crystalline Substances:

1. Have regular arrangement of constituent particles (atoms, molecules or ions). The regular arrangement is in long range order ( almost throughout the crystal).

2. Show anisotropic effect (Different amount or value of the same physical properties in different directions)

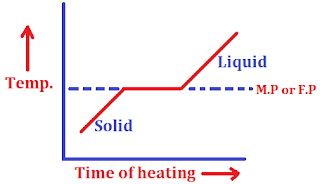
3. Have sharp melting point and fixed enthalpy of fusion. At a particular temperature, crystalline solid converts into liquid.

4. undergo clean cleavage when cut through a sharp object.

5.  Examples of crystalline solids are NaCl, Cu, CaF2, Naphthalene, Diamond, Quartz, Polyethylene.

Amorphous Substances:

1. Disorder pattern of arrangement of constituent particles. Even if regular pattern is found that is in short order. This short range orderly arrangement is equivalent to that of liquid.

2. Show isotropic effect (Same value of the same physical properties in different directions).

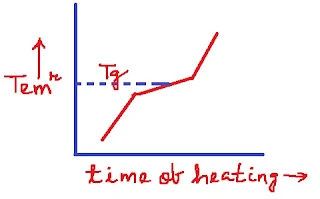
3. No sharp melting point nor any fixed enthalpy of fusion though having a glass transition temperature.

4. undergo irregular cuts when cut through sharp an object.

5. Examples of amorphous solids are: glass, rubber, Teflon, PVC (most of the polymers are amorphous)

Note 1: Some materials may occur both in crystalline and amorphous forms. For example: molten silica (SiO2) on cooling slowly give the crystalline form while cooling rapidly gives the amorphous form.

Note 2: Glass is a super cooled liquid and because of its fluidity, the bottom portion of very old glass windows become thick and appear milky due to partial crystallization.

Note 3: Amorphous silica is the best photovoltaic material available for the conversion of sunlight into electricity.

Classification of solids based on the nature of constituent particles:

a.        Ionic Solids: In these crystalline solids the constituent particles are ions (cations and anions) and strong electrostatic force of attraction exists between them. Example includes Sodium Chloride, Calcium fluoride, Alums. Following are some important characteristics:

·         High melting and boiling points.

·        Act as electrical insulators in solid state and as electrical conductors in aqueous or fused state.

·         Soluble in polar solvents and insoluble in non polar solvents.

·         Hard and brittle.

b.        Molecular solids: In these crystalline solids the constituent particles are molecules. These are further classified into following categories:

·           Non polar molecular solids: Atoms of noble gasses and non polar molecules comes under this category (He, Ne, Ar, H_2, Cl_2, Benzene, CH4, etc.) The type of molecular attractive force is London dispersion forces. The main characteristics of these types of compounds are: Soft, Bad conductor of electricity, low melting and boiling point etc.

·         Polar molecular solids: In these crystalline solids the constituent particles are polar molecules such as HCl, SO_{2, CH3Cl} etc. The type of molecular attractive force is Dipole - Dipole attraction. In general these substances remain in liquid or gaseous state at room temperature. The main characteristics of these types of compounds are: Soft, Bad conductor of electricity, comparatively higher boiling and melting points than non polar molecular solids. The relative intensities of dipole - dipole forces may be represented by the equation:

Where μ1 and μ2 are the molecular dipole moments in Debyes, r is the average distances of separation between two dipoles (angstrom), T in Kelvin, k is Boltzmann Constant.

·   Hydrogen bonded molecular solids: The constituent molecules are held together through hydrogen bonds in these crystalline solids. Example includes soild CO2, H₂O, NH₃ etc. The main characteristics of these types of compounds are: volatile liquids or soft solids at room temperature, bad conductors of electricity, melting and boiling points are generally higher than molecular solids.

c.     Covalent or Network solid: In these crystalline solids the constituent particles are non metal atoms linked each other by covalent bonds throughout the crystal. Since these covalent bonds in these cases are very strong, these are very hard, having high melting and boiling points. Examples are diamond, Silicon carbide (SiC), SiO2 (quartz), AlN, BN, Graphite etc. 

Each carbon atom in diamond is covalently bonded to four neighbours, through sp³ hybrid sigma bonds. The tetrahedral framework extends througout the solid like the steel framework. This structure is the reason for the great hardness of the solid.

The carbon atoms in graphite are sp² hybridized, and the free electrons (in the unhybridized p orbital) are the cause of conduction of electricity. It consists of flat sheets of sp2 hybridized carbon atoms bonded covalently into hexagons. Due to weak bond, two successive sheets (layers) can slide one over another. This is the reason it is used as lubricant at high temperature in heavy machinery.

d.   Metallic Solids: In these crystalline solids the constituent particles are positively charged metal ions (or called the metallic kernel) and free electrons. Since these free electrons can flow, they are called the sea of mobile electrons. These electrons attract the positively charged metal ions around them and hold them together. This type of bond is called metallic bond. Following are some important characteristics:

 ·           High electrical and thermal conductivity.

·           Possess metallic luster.

·           Highly malleable and ductile.

·           Most of the metals have high melting points.

Note: Order of deceasing M.P of various types of solids is: Covalent > ionic > metalic > hydrogen bonded molecular > polar > non polar

Note: Metallic solids show highest conductivity.

Crystal Lattice, Lattice points and Unit cell:

Space lattice and unit cell can be well understood step wise from two dimensions to three dimensions.

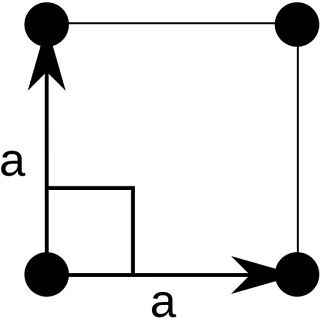
Two dimensional lattices: A two dimensional lattice is a periodic arrangement of points on the plane of paper. Four points are suitably chosen and joined accordingly to obtain a parallelogram called a two dimensional unit cell. These types of unit cells are called as primitive unit cells. When an interior point appears among four suitably chosen points, the unit cell obtained by joining these four points is called a centered unit cell.

           Based on the lengths of sides (a, b) and the angle between them, there are five types of two dimensional lattices:

Square (a = b, angle = 90⁰) (2d primitive tetragonal lattice unit cell),

Rectangular(a ≠ b, angle = 90⁰ ) (2d primitive orthorhombic, rectangular unit cell),

Parallelogram (a ≠ b, angle ≠ 90⁰) (2d monoclinic lattice unit cell),

Rectangular with interior point (2d centred orthorhombic, rectangular unit cell) and 

Rhombus (a =b, angle = 60⁰)

Three dimensional crystal lattices: The three dimensional arrangement (as a three dimensional network) of identical points in the space which represent how the constituent particles (atoms or molecules or ions) are arranged throughout a crystalline substance is called the crystal or space lattice.           

              The positions occupied by the constituent particles (atoms or ions or molecules) of a crystalline substance are represented by points in a crystal lattice are called the lattice points or lattice sites.

            The smallest portion of a crystal lattice which when repeated in different directions, generates the entire crystal lattice is called the unit cell.

Parameters of a unit cells: A unit cell is characterized by its edge lengths or axial lengths (a, b, c) and the angles between the edges or axial angles (α, β, γ). Based on these six parameters there are seven types of unit cells called the seven crystal systems or seven primitive unit cells:

Cubic (a = b = c, α = β = γ = 90⁰ )

Rhombohedral ( or Trigonal) (a = b = c, α = β = γ ≠ 90⁰ )

Tetragonal (a = b ≠ c, α = β = γ = 90⁰  )

Hexagonal (a = b ≠ c, α = β = 90⁰ , γ = 120⁰ )

Orthorhombic (a ≠ b ≠ c, α = β = γ = 90⁰ )

Monoclinic (a ≠ b ≠ c, α = γ = 90⁰  ≠ β )

Triclinic (a ≠ b ≠ c, α ≠ β ≠ γ )

    If we further classify the unit cells of seven crystal system this produces fourteen different lattices called The Bravais lattices. For example the cubic unit cell of the seven crystal system is further divided into three different unit cells, namely simple cubic (the primitive unit cell), body centred cubic and Face centred cubic unit cells.

Calculation of the number of atoms per unit cell: Before calculating the number of atoms per unit cells, let us create an idea about the contribution by a particle to a unit cell present at any position.

Contribution by a particle present at the corner of a cubic unit cell = 1/8 atom

Contribution by a particle present at the centre of a cubic unit cell = 1 atom

Contribution by a particle present at the face of a cubic unit cell = 1/2 atom

Contribution by a particle present at the edge of a cubic unit cell = 1/4 atom

Simple Cubic Unit Cell:  It contains eight atoms (one atom each at every corner). An atom at any corner touches all the atoms at its immediate corners. Thus the coordination number of any particle (the number of its nearest neighbours) is 6.

           The number of atoms per simple cubic unit cell = 8 x 1/8 = 1

Body centred cubic unit cell: Along with atoms at corners, an addition atom is present at the centre of the unit cell. This atom at the centre touches all the atoms at immediate corners. Thus the coordination number of any particle (the number of its nearest neighbours) is 8.

           The number of atoms per BCC unit cell = 8 x 1/8 + 1 = 2

Face centred cubic unit cell: Along with atoms at corners, one atom each is present at the centre of each face of the unit cell. The atom at the centre of the face touches 12 other atoms (4 immediate corners, 4 atoms at the neighboring centres of faces on left and 4 atoms at the neighboring centres of faces on right). Thus the coordination number of any particle (the number of its nearest neighbours) is 12.

           The number of atoms per FCC unit cell = 8 x 1/8 + 6 x 1/2 = 4

Hexagonal Unit cell: In a hexagonal unit cell, corner is shared by 6 unit cells, face is shared by 2 unit cells and the edge is shared by 3 unit cells. Atoms are present at 12 corners, two faces (top and bottom) and three other atoms which direct towards the three alternative vertical faces which are shared by any unit cells.

           The number of atoms per hexagonal unit cell = 12 x 1/6 + 2 x 1/2 + 3 = 6

           In case of compounds, the numbers of different atoms per unit cell gives rise to the formula of the compound.

Ex: 1 In a compound two elements A and B are arranged in cubic structure. Atoms A are at the corners and the B are at the centres of each faces. What is the formula of the compound?

Explanation: An atom at the corners is shared by 8 eight unit cells and contribution made by such an atom to a unit cell= 1/8 atom

Thus number of atoms of A per unit cell = 8 x 1/8 = 1

An atom at the centre of face is shared by two unit cells and contribution made by such an atom to a unit cell = 1/2 atom

Thus number of atoms of B per unit cell = 6 x 1/2 = 3

And the formula of the compound = AB₃

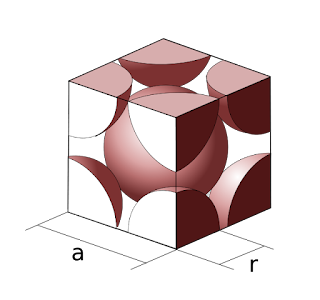
Relationship between edge length (a), nearest neighboring distance (d) and radii of atoms (r):

1.  Simple Cubic Unit Cell: We know that, an atom at any corner touches all the atoms at its immediate corners. Four atoms at four immediate corners are shown in the figure. 

We see that the distance between the two nearest neighbors (d) = the length of edge of cube (a)

 = r + r = 2r.

                                                                        Thus d = a = 2r.

2.    Face Centred Cubic Unit Cell: Three atoms (two at corners and one at the centre of the top face) are shown in the figure.

3.    Body Centred Cubic Unit Cell: In BCC unit cell the atom at the centre of the body touches all the atoms at nearest neighboring corners.

4. Hexagonal Unit Cell: Here, the number of atoms per unit cell is equal to six. Two from 12 corners, one from two face centres and three from the middle layer. 

    As is shown in the figure, the atom present at the centre of the hexagon (in the bottom layer) touches all the atoms at the corners, also each nearby atoms at the corners touch each other. In hexagonal unit cell, the edge length a = b, 

hence  a = 2r = 2 AQ. 

The height of the unit cell is divided into two equal halves. 

Thus height of the unit cell = h = c = 2 OP. 

The atom C present at the centre of the hexagonal (bottom layer), the atom O at the top, the atom A and B touch each other to form a tetrahedron whose configuraion is given below.

Packing fraction and packing efficiency:

            The fraction of total volume of a unit cell occupied by atoms is called the packing fraction and the percentage of total volume of a unit cell occupied by atoms is called the packing efficiency.

1.         Simple Cubic Unit Cell:

Packing fraction = volume occupied by atoms / volume of the unit cell

We have already derived for simple cubic unit cell, a = 2r

Number of atoms per unit cell = 1

Volume of one atom of radius r = 4/3 (π r³)

Volume of the unit cell = a³ = (2r)³ = 8r³

== > Packing fraction = 4/3 (π r³) / 8r³ = π / 6 = 0.524

== > Packing efficiency = packing fraction x 100 = 0.524 x 100 = 52.4%

2.         Body Centred Cubic Unit Cell:

3.        Face Centred Cubic Unit Cell:

4. Hexagonal Unit Cell: 

Example: Xenon crystallizes in FCC arrangement. The edge length of unit cell is 620 pm. What is the nearest neighboring distance and what is the diameter of a xenon atom?

Explanation: 

Example: Cesium bromide crystallizes in BCC pattern. The ionic radii of Cs⁺ and Br^- are 1.88A⁰ and 1.82A⁰ respectively. Determine the packing fraction.

Explanation: 

Try yourself: An element A has BCC structure and guest atoms B, of largest possible size are present at each edge centres without disturbing the original unit cell dimension. Determine the void space of this unit cell.

Density of solids:

            The density of a unit cell of a crystal is equal to the density of the concerned solid. Thus density of the unit cell = Mass of the unit cell / volume of the unit cell.

The mass of the unit cell = number of atoms in the unit cell (represented as Z) x mass of one atom

== > The mass of the unit cell

= Z x (atomic or molecular or formula mass of the particle (denoted as M) / 6.023 x 10²³)

Volume of the cubic unit cell = a³

Thus density of the solid = ZM/ a³N_A, where N_A  = Avogadro’s Number

Ex:4 Aluminium crystalises in a cubic lattice with an edge of 404pm and density of the metal is 2.70g/cm³. Predict the type of unit cell present in aluminiium.

Explanation: We know, density of the solid = ZM/ a³N_A

Atomic mass of Aluminium, M = 27

a³ = (4.04 x 10 ^-8 cm) ³

Thus 2.70 = Z x 27 / ((4.04 x 10 ^-8 cm) ³ x 6.023 x 10²³ ) = 3.097 ≈ 4

Hence Aluminium forms face centred cubic lattice.

Close packing of Solids:

Three dimensional close packing of particles in solids can be understood step wise from one dimensional then two dimensional and finally to three dimensional.

One dimensional close packing: Here particles are packed closely in one direction (in only one axis say X axis). Atoms are arranged in a row touching each other. 

Two dimensional close packing: When particle are packed in two directions (in X and Y axis say) it is called two dimensional close packing. This can be generated by stacking (placing) the rows of closely packed spheres (one dimensional pack of atoms). This can occur in two ways:

a.      Two dimensional square close packing: Atoms in this type are packed in such a way that atoms of one row fall just below the atoms of another row and centers of all consecutive atoms are in a straight line.

b.      Two dimensional Hexagonal close packing: In this packing the atoms are packed in such a manner that the atoms of the second row fit in the depressions of the atoms of the first row.

Three dimensional close packing: Atoms (spheres) are packed in all possible directions (X, Y and Z axis) forming the three dimensional network of the crystal. This can be generated when two dimensional layers of atoms are stacked one above another. Accordingly we can have three types of packing, one from square close pack and two from the hexagonal close pack in two dimensions.

A. The AAA type three dimensional packing from two dimensional square close pack: In this case the spheres (atoms) of the second layer are placed over the first layer in such a manner that the spheres of the upper layer are exactly above those of the first layer. Similarly we can place such layers one above another. Since all layers are aligned similarly, each layer is denoted as A and we get AAA type three dimensional close packing.

The unit cell obtained from this packing is simple cubic. The coordination number is 6 and packing efficiency is 52.4%.

The rest two types of three dimensional packing from two dimensional hexagonal close packing are due to the placing of the third layer of spheres w.r.t. the first layer.

For both of these packing the placing of the second layer over first layer is similar.

The second layer is placed over the first layer such that the spheres of the second layer are placed in the depressions of the first layer. Since the spheres of the two layers are aligned differently, the first layer is marked as A and the second as B.

B. The ABAB type close packing (Hexagonal close pack, HCP): When the third layer of atoms is stacked over the second in such a manner that the tetrahedral voids of the second layer is covered up without affecting the octahedral voids, the spheres of the third layer are exactly aligned with those of the first layer, the ABAB type packing is obtained. The coordination number in this case is 12. The unit cell formed from such packing is called hexagonal unit cell. The number of atoms per unit cell is 6. The packing efficiency is 74%.

C. The ABCABC type packing (cubic close pack, ccp): When the third layer of atoms is stacked over the second in such a manner that its spheres cover the octahedral voids and thus the spheres of the third layer are not aligned with those of either first or second layer. A new layer C is formed and the packing is called ABCABC type packing. The coordination number in this case is 12. The unit cell formed from such packing is FCC unit cell. The number of atoms per unit cell is 4. The packing efficiency is 74%.

Voids or interstitial spaces:

        A tetrahedral void is formed by four atoms (spheres) when four of these atoms (spheres) lie at the vertices of a regular tetrahedron and touch each other. Similarly, an octahedral void is formed by six (atoms) spheres when these atoms lie at the vertices of a regular octahedraon, four of which lie in one plane and touch each other, the fifth atom lies above the plane and the sixth below the plane.

    

The number of tetrahedral voids = 2n

The number of octahedral voids = n     (where n = numbers of atoms per unit cell)

Relationship between radius of the octahedral void (r) and the radius of the atom (R):

From the figure, atoms of radius (R) creating octahedral void of radius (r). The fifth and sixth atomswhich are present above and below the plane of the four atoms are not shown.

ABC is aright angled triangle, 

=> BC² = AB² + AC²     

 => (2R)² = (R+r)² + (R+r)² = 2(R+r)²   

=> 2R² = (R+r)²    

=> √2R = R+r     

=> r = R (√2-1)   

=> r = 0.414R

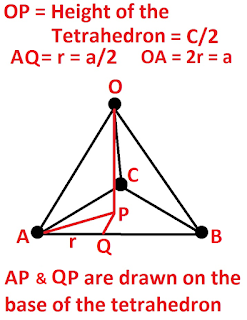
Relationship between radius of the tetrahedral void (r) and the radius of the atom (R):

A tetraherdral void is represented by the figure where three atoms A, E, F form the triangular base and the fourth atom (B) lies at the top. The tetrahedral void comes within these four spheres.

In right angled triangle ABC, AB = √ (AC² + BC²) =  √(a² + a²) = √2a

The spheres A and B are actually touching each other, => AB =2R = √2a

=> R = a/√2   -----eqⁿ 1

In Δ ABD, AD = √ (AB² + BD²) = √{(√2a)² + a²} = √3a

In the body diagonal tetrahedral void is present which touches atoms A and D. 

Thus AD = R+r+r+R

=> AD = 2 (R+r) = √3a => R+r = √3a/2   ----- eqⁿ  2

Dividing eqⁿ 2 by 1, (R+r)/R = (√3a/2 ) / (a/√2) =  √3/√2

=>1 + (r/R) = √3/√2     => r/R =  (√3/√2) -1 => r = 0.225R

Example: A solid crystalises in NaCl type structure. If the radius of the cation A is 100pm then calculate the radius of anion B.

Explanation: In NaCl the anions (in this case B of radius R) occupy the lattice sites and the cation (in this case A of radius r) occupy the octahedral voids. We know r = 0.414R =>  100pm = 0.414R 

=> R =  100/0.414 = 241pm

Try Yourself: A compound is formed by two elements X and Y. Atoms Y occupy the lattice points (or in the ccp arrangement) and the X atoms occupy all octahedral voids. What is the formulas of the compound?

Try Yourself: Two elementts A and B form a compound. Atoms of element B form hcp lattice and the atoms of A occupy 2/3^rd of tetrahedral voids. What is the formula of the compound?

Radius Ratio and coordination number:

    The ratio of the radius of the cation (r₊) to radius of the anion (r_-) is called the radius ratio.

    Mathematically, radius ratio = r₊/r_-

    The co-ordination number of an ion is defined as the number of oppositely charged ions sorrounding it.

    The formula indicates that the larger the size of the cation, greater is the radius ratio and ultimately a higher cordination number. The cordination number can be used to determmine the structure of the crystal. The follwing table lists all these things:

Radius Ratio	C.N	Structure	Structure type	Example
1-0.732	8	Body centred cubic	Caesium chloride	CsI, CsBr, TlBr, TlCl
0.732-0.414	6	Octahedral	Sodium chloride	NaBr, KBr, MgO, MnO, CaO, CaS
0.414-0.225	4	Tetrahedral	Sphalerite, ZnS	CuCl, CuBr, CuI, BaS, HgS
0.225-0.155	3	Planar triangular		B2O3

Note: The above table must born this idea in mind that the minimum size of the octahedral void in which the cation can be fiited so the cation touches all the anions and also all anions making the octahedral voids touch each other = 0.414 x r_- . But as the size of the cation increases the anions which were in contact of each other start separating. The arrangement remains octahederal upto a  maximum size of 0.732 x r_- after which the structure becomes CsCl type.

Example: A compound AB has NaCl type structure. If the cationic radius 75pm what would be the maximum and minimum sizes of the anions filling the voids?

Expanation: NaCl has an octahedral type structure. Radius ratio = r₊/r_- = 0.732-0.414.

Given that r₊ = 75 pm

Minimum value of r_- = r₊/0.732 = 75/0.732 =102.5pm

Maximum value of r_- = r₊/0.414 = 75/0.414 = 181.2pm

Try Yourself: A solid A⁺B^- has a NaCl type structure. If the anion has a radius of 250pm what should be the radius of the cation? Can a cation of radius 180pm be slipped into the tetrahedral site of the sais crystal? Hints: Calculate the radius ratio. Then find whether the value falls in the range of 0.414-0.225.

Example: In a face centred cubic lattice, edge length is 400pm. Find the diameter of the greatest sphere which can be fitted into the interstitial void?

Hints: the octahedral hole is larger than the tetrahedral hole. For FCC, R (radius of atom at lattice points) = a/2√2 = 0.3535a. For octahedral hole the range is 0.732-0.414. Thus the largest radius of octahedral hole = 0.414R’

To be continued. So check regularly. Thank You So Much

Image credit:

Diamond Cubic Animation:

(MarinaVladivostok, CC0, via Wikimedia Commons)

https://upload.wikimedia.org/wikipedia/commons/8/89/Diamond_cubic_animation.gif

Graphite.gif

(Saumitra R Mehrotra & Gerhard Klimeck, CC BY 3.0 <https://creativecommons.org/licenses/by/3.0>, via Wikimedia Commons)

https://upload.wikimedia.org/wikipedia/commons/2/21/Graphite.gif

2D monoclinic unit cell:

By Officer781 - Own work. Edited from File:2d-bravais.svg, CC BY-SA 4.0, https://commons.wikimedia.org/w/index.php?curid=103843539

2D op rectangular orthorhombic lattice:

     By Officer781 - Own work. Edited from File:2d-bravais.svg, CC BY-SA 4.0, https://commons.wikimedia.org/w/index.php?curid=103843574       

2D primitive tetragonal lattice:

By Officer781 - Own work. Edited from File:2d-bravais.svg, CC BY-SA 4.0, https://commons.wikimedia.org/w/index.php?curid=103843637

2d centred orthorhombic, rhombus lattice:

By Officer781 - Own work. Edited from File:2d-bravais.svg, CC BY-SA 4.0, https://commons.wikimedia.org/w/index.php?curid=103843593

2d centred orthorhombic, rectangular:

By Officer781 - Own work. Edited from File:2d-bravais.svg, CC BY-SA 4.0, https://commons.wikimedia.org/w/index.php?curid=103843593

Body Centred Cubic

By Cubique_centre_atomes_par_maille.svg: Cdang (original idea and SVG execution), Samuel Dupré (3D modelling with SolidWorks)derivative work: Daniele Pugliesi (talk) - Cubique_centre_atomes_par_maille.svg, CC BY-SA 3.0, https://commons.wikimedia.org/w/index.php?curid=11106686