Chapter Wise IIT JAM Chemistry questions and solution

 Chapter Wise IIT JAM Chemistry: Questions and Solutions 2005 - onwards

Previous Years IIT JAM Chemistry Questions and solutions:

We are here to help you with previous year question papers and solutions of IIT JAM Chemistry arranged chapter wise from 2005 to the recent one. Some expected important questions can also be found.

You should follow certain rules to crack IIT JAM easily. Find these rules and instructions here.

IIT JAM Physical Chemistry:

Atomic Structure and Quantum Chemistry for IIT JAM

As per the statistics of the previous year question, each year you may find upto 3 questions from atomic structure and quantum chemistry. This includes MCQ, MSQ (multiple select questions) and NAT (numerical answer type).

1. For H - like atoms, the ground state energy is proportional to (IIT JAM 2005):     

A.µ /z²      B.z²/µ       

C.µ z²      D.1/ µ z² 

2a.The electronic wave function for hydrogen atom in the 2s state is given as: 

Ψ ∝ (2-r/a₀) exp (-r/2a₀). Determine the most probable radial distance for the electron in this state and also the position of the node in terms of a₀. (IIT JAM 2006): 

2b. Calculate the wavelength corresponding to the lower energy excitation of an electron confined to a one dimensional box of length 1 nm. (IIT JAM 2006): 

3. Which of the following shows the kinetic energy of the ejected electron versus frequency of the incident photons? (IIT JAM 2007)

4. The normalisation constant A for the wave function,

 Ψ(Φ) = A exp (imΦ) where 0 ≤ Φ ≤ 2π is: (IIT JAM 2007) 

 5. (IIT JAM 2007)

 

6. a. (IIT JAM CHEM 2009)

In Bohr's model of hydrogen like atom with atomic number Z. the angular momentum of an electron (Mass m and charge e) is a non zero integral (n) multiple of h/2π.

The electrostatic attraction exerted by the nucleus on the electron is balanced by the centrifugal force of the electron.

Write the mathematical expression for the above statements. Hence obtain the expression for the radius of r of the electron in terms of e, n and z.

6b. 

7. IIT JAM CHEM 2009: The acceptable valence shell electronic arrangement is: the 

8. An electron is found in an orbital with one radial node and two angular nodes. Which orbital the electron is in?

a. 1s    b. 2p.   c. 3d.    d. 4d (IIT JAM Chem 2009)

9. An atomic orbital is described by the wave function, (IIT JAM 2010)

a. identify the atomic orbital and calculate the mean or the average radius of this orbital  in terms of a₀.

b. Calculate the most probable radius (in terms of a₀.) at which an electron will be found when it occupies this orbital?

10. IIT JAM 2011

In the following equation, X is:

Am(95, 241) + α ----> Bk (97, 243) + X

11. IIT JAM 2011

i. U (92,235)+ n (0,1) -----> A(52,137) + B (40,97) + ______

ii. Se (34,82) ------> 2e(-1,0)+______

12. IIT JAM CHEMISTRY 2012 Q 42.

13. IIT JAM 2013 : For unnormalised wave function, 

Ψ (r, θ, φ) = sinθ cosφ ((2r/a₀) - (r/a₀)²) exp (-r/a₀), the number of radial node is ___________.

14. IIT JAM 2014 : The energy of an electron in a hydrogenic atom with nuclear charge z varies as: 

A. Z         B. Z²       

 C. 1/Z         D. 1/Z² 

15. IIT JAM 2015

Click here get QnA of  IIT JAM 2015

16. IIT JAM 2016 The effective nuclear charge of helium atom is 1.7.  The first ionization energy of helium atom is _________.

A. 13.6                B. 23.1                C. 39.3                D. 27.2

17. IIT JAM 2016 (MSQ): 3pz orbital has:

A. one radial node           B. Two radial nodes

C. one angular node        D. two angular nodes

18.  IIT JAM 2016 (Numerical answer type, NAT) The isotope 84 Po 214 undergoes one alpha and one beta particle emission sequentially to form an isotope  X. The number of neutrons in X is __________.

19.  19. IIT JAM 2016 (NAT) Effective nuclear charge for 3d electron of vanadium according to Slater’s rule is ________.

=> Zeff = z – σ = 23 – 19.7 = 3.3

=> Zeff = z – σ = 23 – 19.7 = 3.3

20. IIT JAM 2017: For a particle in one dimensional  box of length  L with potential energy V(x) = 0 for L > x > 0 and V(x) = infinity for x ≥ L and x ≤ 0, an acceptable function consistent with the boundary conditions is _______. (A and B are constants)

a. A cos (nπx/L)      b. B(x+X²)

c. Cx³ (x-L)               d. D/ Sin (nπx/L)

21. IIT JAM 2018: Which of the following set(s) of quantum numbers is (are) not allowed?

A. n =3, l=2, m = -1

B. n =4, l=0, m = -1

C. n =3, l=3, m = -3

D. n =5, l=3, m = +2

22. III JAM Chem 2018 NAT: The electron of a hydrogen atom is in its nth Bohr orbit having de-broglie wavelength of 13.4 angstrom. The value of n is _____. (radius of nth orbit = 0.53 n^2 Angstrom)

23. III JAM Chem 2019 NAT: Ionisation energy of hydrogen atom is 13.6 ev and the first ionization energy of sodium atom is 5.1ev. The effective nuclear charge experienced by the valence electron of sodium atom is _____.

24. The Bohr’s first orbit radius of hydrogen is 53 pm. Radius of third Bohr’s Orbit radius is ___.

25. III JAM Chem 2020 (NAT):  The longest wavelength of light absorbed by hydrogen like atom is 2.48 nm. The z = ____. (Rydberg constant = 109700 cm^-1)

Answers 

Ans. 1. The formula for energy of electron in an orbit is 

E_n = - me⁴ z²/8ε₀² n² h²   

replacing mass by reduced mass the formula becomes 

E_n= - µe⁴ z²/8ε₀² n² h². 

Thus the answer is  C. µ z² 

2a and 2b. Click here to get the answer of 2a. And 2b.

If you want to test yourself whether you are for IIT JAM Chemistry, then appear in these Online Mock Test.

3. The incident photon should have some energy called work function below which no electrons can be ejected. The energy with which electron is bound to the surface is called the work function and the corresponding frequency is called threshold frequency (𝜈0). According to Einstein's Photoelectric effect,

K.E = h𝜈 - h𝜈0 

This equation is equivalent to: y=mx-C. Hence the correct option should be: A

If you want to know hidden information about Nobel prize in chemistry then click here.

4. Click here to get the answer.

5. According to the question, overlap between dzx and pz orbital is positive as both have the same sign (+ and -) to the same side. Hence they are ready for the bond formation. Thus the answer should be A.
6. Click here to get the answer 

6.b. Two answers respectively are ₈ O ¹⁷ and ₄ Be ⁸

7. The answer should be c. as all unpaired electron are having similar spin.

According to Hund's rule, no two electrons can occupy same spin direction in same orbital. Orbitals of a subshell are filled up first with similar spin then filled up with opposite direction during pairing.

Also maximum spin multiplicity and Large L value is stable configuration.

8. The formula of angular node = l That means the angular nodes of an orbital is equal to it's Azimuthal Quantum Number.

Since s orbitals have l = 0, they have no angular nodes. p and d orbitals have 1 and 2 angular nodes respectively.

Thus the answer should either be 3d or 4d.

Now the formula of radial nodes = n-l-1. Since 4-2-1=1 and according to the question, the radial nodes = 1, the orbital should be 4d. Thus the answer should be d.

9. -Since the wave function only depends on r, it can be represented as:

Ψ(r, θ,Φ) = Ψ(r, 0, 0) . Thus it has no angular nodes (as it is independent of  θ and Φ ). Hence it is an s-orbital.

Thus the given orbital is 1s (n,l,m = 1,0,0). The average radius of the orbital can be found as follows

b. The most probable radius of the electron in 1s orbital can be found as follows:

Thus the given orbital is 1s (n,l,m = 1,0,0). The average radius of the orbital can be found as follows

Thus the average distance is 50% greater than the most probable distance of the electron.

10. In the given reaction, Am stands for Americium having atomic number 95 and mass number 241, alpha particle has a charge of +2 and mass of 4. It means when Am is bombarded with alpha particle the total nuclear charge becomes 97 and mass becomes 245 on the left. Since Bk stands for Berkelium, the particles emitted should be two neutrons 2n. Thus X stands for 2n (0,1).

11. Similar as above

13. According quantum mechanics, the atomic orbitals can be written by the wave function Ψ (r, θ, φ) which is obtained by solving Schrodinger wave equation. That means a function of three polar coordinates r, θ and φ can be represented as a product of three independent wave function  which depend separately on r, θ and φ respectively.

Ψ (r, θ, φ) = R(r) . Θ (θ) . Φ (φ)

Radial function R(r) gives the distribution of the electron as a function of  the distance r from the nucleus. The R(r) part of the complete wave function can be isolated looking into the r realated part. in this case, 

R(r) = ((2r/a₀) - (r/a0)²) exp (-r/a₀)

All points for which r = 0 gives the radial nodes.

When R(r) = 0,

((2r/a₀) - (r/a₀)²) exp (-r/a₀) = 0

=> r/a₀ (2- (r/a₀)) = 0

=> r =0 and r = 2a₀

=> number of radial nodes = 2

14. Energy of an electron in Bohr's orbit = -kZ²/n², thus the answer should be B.

16. Energy of an electron  = -kZ²/n²

= - 13.6 Z²/n²

Here effective nuclear charge (Z*) is given that can be put into the above formula for Z as because only two electrons are present in helium atom.

This energy as calculated by the formula should be equal to the ionization energy as this energy when given to the electron it is ejected.

Ionisation Energy of an electron = -13.6 (1.7)²/ 1² = 39.3

If you want to know some unknown facts about Nobel Chemistry click here.

17. The number of radial nodes of an orbital = n - l - 1 and the number of angular nodes = l, where n and l are the principal and azimuthal quantum numbers respectively.

3pz orbital has n = 3 and l = 1, hence it should have one radial nodes and one angular nodes. hence the answer should be A and C.

18. On emission of one alpha particle (2 He 4) atomic number reduced by 2 and mass by 4. Similarly on the emission of a beta particle (-1 e 0) only the atomic number is increased by 1.

Thus the mass number of X = 214 – 1x 4 = 210

And the atomic number of X = 84 – 1 x 2 + 1 = 83

Thus the number of neutrons = 210 – 83 = 12719. V has z = 23

₂₃ V = 1s², 2s², 2p⁶, 3s², 3p⁶, 3d³, 4s²

According to Slater’s rule (find it along with periodic classification of elements), the electronic configuration should be written as:

₂₃ V = (1s)², (2s 2p)⁸, (3s, 3p)⁸, (3d)³, (4s)²

σ = Shielding constant for s orbital = number of n shell electrons x 0.35 + number of n -1 shell electrons x 0.85 + number of all other electrons [(n-2) (n-3) or lower shells] x 1 = 1 x 0.35 + 11 x 0.85 = 10 x 1 = 19.70

=> Zeff = z – σ = 23 – 19.7 = 3.3

20. The function is continuous between X = 0 to L and hence the function should be zero at x = 0 and x = L.

Both these conditions are satisfied by the function C. Cx^3 (x-L)

21. B. and C. are not possible as in B. when l = 0, m must be zero but given m = -1 which is not possible. In C. n and l can’t be same as per quantum numbers.

22. 2πr = nλ

=> 2 x 3.14 x 0.53 n² / n.13.4

=> n = 13.4 / 2 x 3.14 x 0.53

=> n = 4.03 = 4

23. Na = (1s)², (2s,2p)⁸, (3s)¹

 Zeff for s electron = z – σ = 11 – (8 x 0.85 + 2 x 1) = 2.2

24. Radius of nth orbit = ao (n²/z)

For hydrogen atom, r = ao (n²/1)

= 53 x (3²)

R = 53 x 9 = 477 pm

25. For longest wavelength, the energy absorbed for the transitiuon should be lowest. Thus the transition referes to 1st to 2nd orbit.

IIT JAM Questions on Gaseous State:

 (JAM 2005) :  Justify the following:

Considering CO2 as an ideal gas, equipartition theorem predicts its total energy as 6.5 kT.

Justification: It’s a linear molecule having 3 numbers (N) of atoms. It has 3 modes of translational, two modes of rotational and 3N-5 modes of vibrational degrees of freedom. Thus according to equipartition principle (each mode contributes ½ kT per molecule or ½ RT per mole), Total energy E(total) = 3/2RT + RT + (3N-5)RT = 6.5 RT per mole or 6.5kT per molecule. Click here to find details about degrees of freedom and equipartition principle.

(JAM 2005): For the distribution of molecular velocities of gases, what is the correct order most probable (m.p), root mean square (rms) and average velocity (avg)?

Ans: mp : avg : rms = (2RT/M)^1/2 : (8RT/πM)^1/2 : (3RT/M)^1/2.

= 1 : 1.128 : 1.224. Thus the order should be: mp < avg < rms

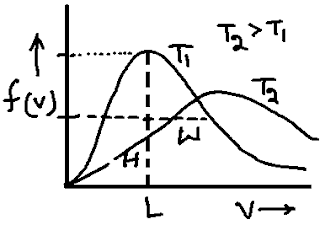
Jam (2006): In the Maxwell distribution of molecular velocity given below as a graph, H is the height of the peak, L is the location of maximum and W is the width at half height. 

What happens to these H, L and W as temperature is decreased:

Ans: 

As the temperature decreases H increases, L and W decreases.

Jam (2007): The Maxwell distribution function for the distribution of speeds of molecules in gaseous system is,

f(c) = 4π(m/2πkT)^3/2 C² exp (-mC²/2kT)

Show that the most probable speed is (2kT/m)^1/2

Proof: The following proof is from the equation,

f(c) = dNc/N = 4π (m/2πkT)^3/2 C² exp (-mC²/2kT)

   The velocity with which most of the molecules move in a container at a particular temperature is called the most probable velocity.

    We can see from the Maxwell distribution curve that the most probable velocity is the peak of the curve where the value of the derivative must be zero. Accordingly the derivative of dNc with respect to dc must be equal to zero.

(JAM 2008):  Given that the most probable speed of oxygen gas is 1000 m/s, the average speed under the same conditions will be ______?

Ans: C_mp = (2RT/M)^1/2 = 1000 ms^-1

C_avg = (8RT/πM)^1/2 = (4x2RT/πM)^1/2

=> C_avg = (4/π) ^½ x (2RT/M)^1/2

=> C_avg = 2000/1.772 = 1128.6 ms^-1

(JAM 2009):  Write the expression for the vibrational contribution to the total energy of CH₄ (g) at 500k. All the vibrational modes are active at this temperature. Also calculate the total internal energy of 1 mole of the gas at this temperature. Click here if want to know details about degreesof freedom and equipartition principle.

Ans: Vibrational degrees of freedom are active at high temperature. CH₄ (N = 5, as it has 5 atoms) is a non linear molecule. Thus the number of vibrational modes = 3N-6 =9.

According to equipartition principle each mode (degrees of freedom) contributes 1/2RT to the total energy but since vibrational mode includes both potential and kinetic contributions, each vibrational mode contribute 2x(1/2RT) or simply RT.

Thus vibrational contribution to the total energy = 9 RT = 9x8.314x500 =37400 J/mole = 37.4kJ/mol

Total internal energy = translational contribution + rotational contribution + vibrational contribution

Any molecule has 3 translational degrees freedom. CH₄ being a non linear molecule has 3 rotational degrees of freedom and as calculated it has 9 vibrational degrees of freedom.

=> I.E = 3x(1/2RT) + 3x(1/2RT) + 9RT = 48.88kJ/mol

(JAM 2010): The molar internal energy of a gas at temperature is U_m(T). The molar internal energy at T = 0 is U_m(0). The correct expression that relates these two with appropriate contributions is:

a) U_m(T) = U_m(0) +3RT (Linear molecule; translation only)

b) U_m(T) = U_m(0) +(5/2)RT (Linear molecule; translation and rotation only)

c) U_m(T) = U_m(0) +(3/2)RT (Non-linear molecule; translation and rotation only)

d) U_m(T) = U_m(0) + RT (Non-linear molecule; translation only)

Ans:

Molar heat capacity, C_V = (∂U/ ∂T)

=>∂U = C_V ∂T

=> ΔU_m = C_V ΔT

=> U_m(T) - U_m(0) = C_V (T – 0)

=> U_m(T) - U_m(0) = C_V T

For linear molecule, C_V = (5/2)R

=> U_m(T) - U_m(0) = (5/2)RT

Also, a linear molecule has 3 translational and 2 rotational degrees of freedom (if temperature not very high, where vibrational mode is not active, as per the options/choices of answer available). Each mode contributes (½)RT. Thus the option b is correct.

(JAM 2011): (a)Same as (JAM 2007)

(b) Calculate most probable velocity of CH₄ at 127⁰_c. (R = 8 J K^-1mol^-1)

Ans: M = 16 = 16X10^-3 kg/mol

T = 127⁰_c = 400k

V_mp = (2RT/M)^1/2

= [(2x8x400)/ 16X10^-3]^1/2

= 632.45m/s

(JAM 2012)(i) Show that for n moles of a van der Waal’s gas:

 (∂U/∂V)_T =n²a/V²

(ii) can a gas that obyes the equation of state p(V-nb) = nRT be liquefied. Explain.

(JAM 2013): No questions particularly on gaseous state.

(JAM 2014): According to equipartition principle of energy the molar heat capacity at constant volume for CO­₂ , SO₂ and H₂O gas follows the trend:

Ans: CO­₂ is a linear molecule where as the rest two are nonlinear molecules.

For linear molecules, and according to equipartition principle of energy,

Internal energy (U) = Contribution from Translational + Rotational + Vibrational DOF

Linear molecules with two atoms (N=2) follow,

U = 3X(1/2)RT + 2 X(1/2)RT  +  (3N-5)RT

=6.5RT

Thus C_v = (∂U/∂T)v = (∂/∂T) 6.5RT = 6.5R

Non-linear molecules with three atoms (N=3)  follow,

U = 3X(1/2)RT + 3 X(1/2)RT  +  (3N-6)RT

=6RT

Thus C_v = (∂U/∂T)v = (∂/∂T) 6RT = 6R

Hence order of molar heat capacity at constant volume will be:

CO₂ > SO₂ = H₂O

(JAM 2015): In an ideal monoatomic gas, the speed of sound is given by (5RT/3M)^1/2. If the speed of sound in argon at 25⁰c is 1245 km hr^-1, the root mean square velocity in ms^-1 is __________.

Ans: RMS = (3RT/M)^1/2

1245 km/hr = 1245 X 1000 m/hr

= (1245 X 1000) m/3600 sec

= 345.833 m/s

Given , speed of sound = (5RT/3M)^1/2

= 345.833 m/s

= (5) ^½ . 3 (3RT/M)^1/2 = 345.833 m/s

RMS = (3RT/M)^1/2

= 463 m/s

(JAM 2016): A 10 ltr flask containing 10.8 g of N₂O₅ is heated to 373 k, which leads to its decomposition according to the equation

2 N₂O₅  -------> 4NO₂   + O₂. If the final pressure in the flask is 0.5atm, then the partial pressure of O₂ in atm is _______.

Ans: 

(JAM 2016): The relationship between the van der Waals b coefficient of N₂ and O₂ is ______.

Ans: The van der Waals constant b represents excluded volume (from the total volume of the vessel) of a gas. The greater the size of the molecule greater is the excluded volume.

The size of N₂ is 300 pm compared to 292 pm in O₂. Thus b(N₂) > b(O₂)

(JAM 2016): From the kinetic theory of gases, the ratio of most probable speed to root mean square speed is _______.

Ans: C_mp/C_rms = (2RT/M)^1/2 / (3RT/M)^1/2 = 2^1/2/3^1/2

(JAM 2017): A vessel contains a mixture of H₂ and N₂ gas. Density of the mixture is 0.2g L^-1 at 300k and 1 atm pressure. Assuming that both the gases behave ideally, the mole fraction of N₂ in the vessel is ______.

(JAM 2017): The number of normal modes of vibrations in naphthalene is _______.

Ans: Naphthalene is a non-linear molecule with 18 number (N) of atoms.

Normal modes of vibrations = 3N -6

=3x18 – 6 = 48

(JAM 2018): The value of C_v for 1 mole of N₂ gas predicted from equipartition principle, ignoring vibrational contribution is ________.

Ans: N₂ is linear diatomic (N = 2) molecule.

Cv = 3X(1/2)R + 2X(1/2)R

=(5/2)R = (5/2)x8.3

=20.75Jk^-1mol^-1

(JAM 2018): Assuming ideal gas behavior, the density of O₂  gas at 300 k and 1 atm is ______.

Ans: We know PV = nRT

=> P = (n/V)RT

=> P = (w/mV) RT

=> Pm = dRT

=> d = Pm/RT

Putting the values,

Density = 1.30gL^-1

(JAM 2018): The behavior of Cl₂ is closest to ideal gas at ______ temperature and ______ pressure.

Ans: high temperature and low pressure

(JAM 2019): The total number of degrees of freedom of HBr molecule that is constrained to move in a straight line but does not have any constraints on rotational and vibrational degrees of freedom is ­­­­­­­­­­______.

Ans: Linear diatomic (N =2)  molecule. One translational + 2 rotational + 1 vivrational DOF. In total 4.

(JAM 2019): The ratio of RMS velocity for molecular oxygen and hydrogen is ________.

Ans:  RMS velocity = (3RT/M)^1/2

RMS of O₂ / RMS of H₂

= (M_H2/M_H2)^1/2

= 1:4

(JAM 2020): The Boyle’s temperature (T_b) is the temperature at which the properties of a real gas coincide with those of an ideal gas in the low pressure limit. What should be the graph for Z (compressibility factor) versus pressure at T_b?

(JAM 2021): If the root mean square velocity of hydrogen at a particular temperature is 1900 ms^-1, then the rms speed for nitrogen at the same temperature should be ____.

Ans: Apply the formula RMS velocity = (3RT/M)^1/2 for both the gases and take the ratio to cancel out similar terms. Find answer next.

IIT JAM Questions on Liquid State:

(JAM 2005): At 20 ⁰c the vapour pressure of two pure liquids X and Y which form an ideal solution are 70 torr and 20 torr respectively. If the mole fraction of X in solution is 0.5, find the mole fractions of X and Y in the vapour state.

Solution: Applying Raoult’s law of vapour pressure,

P_X = P⁰_X . X_X = 70 X 0.5 = 35torr ----------eq. 1

[X_X = molefraction of X in the liquid state]

P_y = P⁰_y . X_y = 20 X 0.5 =10 torr ----------2

P_T = 35 + 10 = 45 torr --------------3

Applying Dalton’s law of partial pressure,

P_X = P_T . y_X ------------4

[y_X = molefraction of X in the vapour state]

P_y = P_T . y_y   ---------------5

Using eq. 1, 3 and 4, we get,

And y_y  = 1 – 0.77 = 0.22

No particular question in IIT JAM Chemistry 2006 from Liquid state.

JAM (2007):

No particular question in IIT JAM Chemistry 2008 and 2009 from Liquid state.

(JAM 2010): At room temperature, HCl is a gas but HF is liquid, because:

Ans: Due to intermolecular H-Bonding in HF

(JAM 2010): Addition of 1 g of a compound to 10 g water increases the boiling point by 0.3 ⁰ c. The amount of compound needed to prepare 500 ml of 0.1M solution is: (given that no dissociation or association of solute takes place, Kb of water = 0.513 k kg mol^-1) 

(JAM 2011): The most viscous liquid among water, methanol, ethylene glycol and glycerol is:

Ans: Glycerol having 3 –OH groups makes maximum intermolecular hydrogen bonding. It is the most viscous among given liquids.

(JAM 2011): If 4 moles of MX₂ salt in 1 kg water raises boiling point of water by 3.2 k, calculate the degree of dissociation of MX₂ in the solution. (Kb = 0.5 K Kg mol^-1)

Solution: if association or dissociation of solute particles take place then we have the formula, ΔTb = i Kb m, where i = van’t Hoff factor.

Thus, 3.2 = i X 0.5 X 4

=> i =1.6

The relation between i and  α in case of dissociation of solute is :

i = 1 + α (n-1) and MX₂ gives one M ²⁺ and two X^- ions number of particles after dissociation, n = 3. Putting the value of n and i in the equation we get α = 0.3

(JAM 2012): An aqueous solution containing 1g L^-1 of a polymer exerts osmotic pressure of 4 torr at 300 K. The molar mass of the polymer is:

Ans: Osomotic pressure = π = 4 torr = 4 / 760 atm

Corresponding R = 0.082 L atm K^-1 mol^-1

Π = wRT/mV

=> m = wRT/ Π V

 = (1 X 0.082 X 300) / (4/760) X 1

= 4674g/mol

(JAM 2012): The molality of (NH₄)₂SO₄ solution that has same ionic strength as 1 mol kg^-1 of KCl is:

Solution: 

(JAM 2012): Consider ideal mixing of 2 moles of toluene and 2 moles of benzene at 1 atm and 300 K. Calculate the values of ΔV_mix , ΔU_mix , ΔH_mix , ΔG_mix , ΔS_mix for the process. (ln 2 = 0.69).

Solution: An ideal solution can be considered as a mixture of two ideal gasses.

Also for ideal solution, each of ΔV_mix , ΔU_mix , ΔH_mix isequal to 0

The initial pressure of each gas = 1 atm and temperature = 300K

Each of the gas is of 1 mole. Hence the mole fraction (χ) of each component = χ = 2/ (2+2) = 0.5

The Gibb’s energy of mixing,

ΔG_mix = nRT (χ₁ ln χ₁ + χ₂ ln χ₂)

= 4 X 8.314 X 300 [0.5 ln(1/2) + 0.5 ln(1/2)}

= 4 X 8.314 X 300 [ ln(1/2)]

= - 6.916 KJ

The negative value of free energy of mixing indicates the spontaneous process while two liquids are added.

ΔS_mix = ( - ΔG_mix) / T

= - (- 6.916) / T

= + 6.916 / 300 = 23.05 j/K

See details below:

https://chem.libretexts.org/Bookshelves/Physical_and_Theoretical_Chemistry_Textbook_Maps/Supplemental_Modules_(Physical_and_Theoretical_Chemistry)/Thermodynamics/Ideal_Systems/Thermodynamics_of_Mixing

(JAM 2013): No particular question from liquid state.

(JAM 2014): Mixed question (Change in enthalpy, free energy, osmotic pressure rate, constant, heat capacity versus temperature)

Solution: (I - E) Enthalpy of the reactants is greater than zero and the possible curve should be one of B, C and E. Here number of moles of products is greater than the number of moles of reactants. Δn_g = +Ve. Change in enthalpy is an extensive property, hence increases as the number of moles increases. Thus the curve E fits to this (i). 

(II - D) Osmotic Pressure of ideal solution (π), is directly proportional to the temperature. Hence Curve D is the right choice.

π V = nRT

(III - B) Standard free energy of formation (Δ_fG⁰) of elements is taken as zero. Δ_fG⁰ versus temperature is constant. Hence Curve B fits into it.

(IV - C) The molar heat capacity at constant volume for an ideal gas as predicted by equipartition of energy is given by, C_v.m = (∂ U_m / ∂ T). Thus molar heat capacity is inversely proportional to the temperature. Curve C fits into it.

(V - A) Arrehenius equation,

K = A Exp (-E_a/RT)

Thus k ∝ Exp (-1/T) and the Exponential Curve A fits to it.

(JAM 2014): The ionic strength of 0.1M aqueous solution of Fe₂(SO₄)₃is ______.

Soution: Refer corresponding JAM 2012 Q and A.

 (JAM 2015): No particular question from liquid state.

(JAM 2016):  

Cu(s) + 4H+(aq) +2NO3- (aq) -----------> 2NO2 (g) + Cu2+(aq) +2H2O  

In the above reaction, at 1 atm and 298 K, if 6.36g of Cu is used. Assuming ideal gas behavior, the volume of NO2 produced in litre is __________. 

Solution: Number of moles of Cu = 6.36/63.6 = 0.1 

from the equation, 

0.1 mole Cu equivalent to 0.2 mole of NO2 

V = nRT/P = (0.2X0.0821X298)/1 = 4.89 L  

 (JAM 2017): No particular question from liquid state. 

(JAM 2018): The volume of 0.3M ferrous ammonium sulphate solution required for the completion of redox titration with 20 ml of 0.1M potassium dichromate solution is _______. 

Solution: In the redox reaction Cr2O72- oxidises ferrous (Fe2+) ion, by the following reaction, 

Cr2O72- + 6Fe2+ + 14H+ ----> 2Cr3++ 6Fe3+ + 7H2O 

Fom the equation,  

1 mole of Cr2O72- requirres 6 moles of Fe2+ 

Number of moles of Cr2O72- (as per the question) = 20 X 0.1 = 2 moles 

Number of moles of Mohr’s salt (Fe2+) ions needed = 6X2 = 12 moles 

Volume of Mohr’s salt required = 12/0.3 = 40 ml 

Alternatively,  

One  mole (n1) of Cr2O72- requirres 6 moles (n2) of Fe2+ 

n1M1V1 = n2M2V2 

=> 1 X 0.3 X V1 = 6 X 0.1 X 20 

=> V1 = 40 ml

(JAM 2019): Assume that the reaction of MeMgBr with ethylacetate proceeds with 100% conversion to give tert-butanol. The volume of 0.2M solution of MeMgBr required to covert 10 ml of a 0.025 M solution of ethylacetate to tert-butanol is ____ ml.

Solution: This question can be solved according to the milimoles of reactant species. 

Milimoles = Molarity X Volume in ml 

Also Volume in ml = Milimoles / molarity 

In a balanced equation moles of reaction can be replaced by milimoles.

 (JAM 2020): The mean ionic activity coefficient for a 0.01 M aqueous solution of Ca3(PO4) 2 is __________.  

[log Y± = -0.059 z+ I Z-I (I1/2 ) ] 

Solution: See IIT JAM 2012 in liquid section.

IIT JAM Questions on Solid State

(JAM 2005): No questions from solid state.

(JAM 2006): No questions from solid state.

(JAM 2007): No questions from solid state.

(JAM 2008): No questions from solid state.

(JAM 2009): No questions from solid state.

(JAM 2010): The structure of rock salt is_________.

Ans:

Tem5psu, CC BY-SA 4.0 <https://creativecommons.org/licenses/by-sa/4.0>, via Wikimedia Commons

https://commons.wikimedia.org/wiki/File:NaCl-layers.png

Vectorization:  GKFX, CC BY-SA 3.0 <http://creativecommons.org/licenses/by-sa/3.0/>, via Wikimedia Commons
https://commons.wikimedia.org/wiki/File:NaCl-Ionengitter.svg

In most of ionic crystal the anions take the lattice point positions and the cations occupy the voids formed by the anions. 

The ionic radius ratio (ratio of radius of cation to the anion is 0.52 which is in between 0.414 and 0.732 suggests an octahedral void)

In NaCl crystal Cl^- ions at lattice points (corner + face centres in FCC or ABCABC arrangement) form the octahedral voids which are occupied by Na⁺ ions. Thus Coordination number of both the ions are =6:6 or 1:1

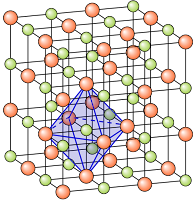
(JAM 2010): Draw a properly labeled unit cell diagram of CsCl. Show through calculations that there is only one CsCl per unit cell.

Ans: 

Chegg Study, CC BY-SA 4.0 <https://creativecommons.org/licenses/by-sa/4.0>, via Wikimedia Commons,  https://commons.wikimedia.org/wiki/File:Struktura_cezijevega_klorida.png

The radius ratio of CsCl is 0.93 suggesting a cubical void or BCC packing and thus ration of coordination number is 8:8 or 1:1.

Number of Cs⁺ ion per unit cell = 1 (at the body centre cubic unit cell)

Number of Cl^- ions present at all corner of Body centre cubic = 8X1/8 =1

Thus there is only one CsCl per unit cell.

(JAM 2011): The oxide that has inverse spinel structure is:

i.FeCr₂O₄          ii.MnCr₂O₄        iii. CoAl₂O₄       iv. Fe₂CoO₄

Solution: Class of minerals of general formula AB₂X₄, having cubic (isometric) crystal system with X anions arranged in cubic close packed lattice and cations A and B occupying some or all of the tetrahedral and octahedral voids are called spinels. Oxide spinels have general formula AB₂O₄, for example, MgAl₂O₄. In the close pack array of anions (e.g., oxide ions), A site cations fill the 1/8 part of the tetrahedral voids and B site cations fill ½ of the octahedral voids.

Its very clear that in a unit cell of FCC the number of atoms at all lattice points = 8X1/8(from corner) + 6X1/2(from face centres) = 4

Hence number of Oxide ions = n = 4

Number of tetrahedral holes = 2n = 8

1/8 th of 8 = 1 = A

Number of octahedral holes = n = 4

½ of octahedral holes = 2 = B

½ of octahedral holes = 2 = B

Hence AB₂O₄ is the formula of normal spinels. For more clarity A can be understood as divalent cations and B can be understood as trivalent cations.

Inverse spinels are closely related to spinels, where the close pack array of anions remain the same but A site ions and ½ of B site ions switch their positions, giving a formula B(AB)O₄. For more clarity, in an inverse spinel the A²⁺ ions occupy ¼ th of octahedral voids along with ½ of B³⁺ ions whereas the other half of the B³⁺ ions occupy 1/8^th of the tetrahedral voids.

In the given question, Fe^III(Co^IIFe^III)O₄ is the inverse spinel because the Co²⁺ ion is octahedrally surrounded.

CFSE of Co²⁺ = d⁷ = t_2g⁵e_g² = (-0.4X5 + 0.6x2) Δ_o = -0.8 Δ_o. This is highest compared to Fe^III  having CFSE 0. Thus Co²⁺ will be stabilized in octahedral voids. This should be the answer.

JAM 2011: The transition metal monoxide that shows metallic conductivity is: i.NiO   ii.MnO    iii.TiO    iv.CoO

Solution: NiO, becaude Nickel can exists both in +2 and +3 oxidation states. The +3 state of Nickel act as a hole and accept electrons from nearby +2  oxidation state to conduct the electricity.

JAM 2012: Draw the unit cell structure of NaCl. Calculate the limiting radius ratio of any ionic solid having NaCl like structure.

Solution: See JAM 2010 for the structure of NaCl

JAM 2013: A hypothetical element (atomic weight 300) crystalises in simple cubic lattice. The first order X-ray diffraction with wavelength 5 A⁰ appears at an angle 30⁰. The density of the crystal is _______ g cm^-3.

Solution: From Bragg’s equation, 2dsin θ = nλ , for first order n=1, Thus 2dsin30⁰=1X5

2d(1/2)=5

d=5 A⁰ = 5X10^-8 cm

d=a (for simple cubic lattice)

density = ZM/a³Na

For simple cubic lattice the number atoms per unit cell z = 1

Na = 6.02X10²³ and M = atomic mass = 300

We got density = 3.985 g cm^-3

For limiting radius Click here.

To be continued.....