Notes on Chemical Kinetics

 Notes on chemical kinetics for intermediate and B.Sc.

The most important part of geting success in any branch of science is to get a quality notes on any topic. In fact, Notes are not mere the duplicate copy of any books, rather they are the systematic arrangements of various important concepts, and problem solving to feed the understanding.

For your best result we are also providing notes on Thermodynamics, Periodic table, Gaseous state, Phase rule, Industrial chemicals and environment, and Solid state.

Now you scroll down for Notes on Chemical Kinetics.

What happens in a chemical reaction?

In a chemical reaction, some or all of the bonds of the reacting species are broken and new bond(s) are formed between different atoms of the same reacting species to form the products. Thus depending upon the nature of bonds of the reactants (whether strong or weak), a reaction may be faster or slower. Clearly, if the bonds of the reacting species are strong, then higher amount of energy is required to break those bonds and the reaction becomes slower as compared to a reaction in which the bonds of the reacting species are weak.  In this regard, the fastness or slowness of a chemical reaction is mathematically termed as the rate of the chemical reaction.

Chemical kinetics is the branch of chemistry which deals with the study of the type, the rate, the factors affecting the rate and the mechanism (molecular events occurring during the chemical reaction) of a chemical reaction.

Rate of reaction (ROR): The change in concentration (M or mol per litre) of any reactant or product per unit time, per unit stoichiometric coefficient in a chemical reaction is called the rate of reaction.

Mathematically,

(ROR) = Change in concentration of any reactant or product/(Change in time . 𝛎)

Where 𝛎 is the stoichiometric coefficient.

Thus the unit of rate of reaction will be M/sec 

or mol L^^-1 sec^^-1 

or mol L^-1 min ^-1

For any gaseous reaction:

We have, PV = nRT     

=> P = (n/V) RT = CRT = [Gas]RT

Thus if temperature is kept constant, [Gas] α partial pressure of the gas

This implies that, in a gaseous reaction the rate can be expressed in terms of partial pressure of gasses.

ROR = Change in partial pressure of any reactant or product / (time taken X stoichiometric coefficient of concerned reactant or product)

Accordingly, the unit of rate of reaction will be atm/sec 

or atm/min 

or bar/sec 

or Pascal/sec etc.

Based on the consideration of time interval we can have two different rates of reaction:

Average rate of reaction: The rate of reaction measured and calculated for a finite time interval (finite time interval,  Δt= t₂ – t₁) is called the average rate of reaction.

Consider a reaction, A(g) → B(g) in which the concentrations of A and B changes with time as follows:

Time in minutes	A (in mol L-1)	B (in mol L-1)
0	1.5	0
5	1.35	0.15
10	1.25	0.25

The stoichiometric coefficients of both A and B in the above reaction are one. Thus if we consider the concentrations of A and B at time 5 minutes and 10 minutes, we have,

ROR(avg)= – Δ[A] / Δt 

= Δ[B] / Δt. 

= –(1.25 – 1.35)  / (10 –5) 

= (0.25 – 0.15) / (10 –5) 

= 0.02 mol L^-1 min^ –1

Note: A negative sign is multiplied while calculating rate of reaction w.r.t. the reactant to get a positive value because rate of reaction can’t be negative also we can say the negative sign indicates a decrease in concentration.

Consider another reaction, 

2A → 3B, for which the change in concentration during the course of reaction is as follows:

	A	B
At t = 0	a M	0
At any time t	(a – 2x) M	3x M

The Stoichiometric coefficients for A and B (νA and νB) in the above reaction are 2 and 3 respectively. Thus,

ROR = – Δ[A] / (Δt. νA) 

= Δ[B] / (Δt. νB) 

= – [(a – 2x) - a] M / (t – 0) min . 2

= [3x – 0] M / (t – 0) min . 3

Instantaneous  rate  of  reaction:  Rate  of  reaction  measured  at  a  particular  instant of The Stoichiometric coefficients for A and B (νA and νB) in the above reaction are 2 and 3 respectively. Thus,

ROR = – Δ[A] / (Δt. νA) 

= Δ[B] / (Δt. νB) 

= – [(a – 2x) - a] M / (t – 0) min . 2

= [3x – 0] M / (t – 0) min . 3

Instantaneous  rate  of  reaction:  Rate  of  reaction  measured  at  a  particular  instant of time  is  called  the instantaneous rate of reaction. The average rate of reaction becomes equal to instantaneous rate of reaction when the time range (Δt) tends to zero.

=>Instantaneous rate of reaction = d[A] / (dt . ν), 

where d[A] and dt  are instantaneous (infinitesimal) change in concentration of reactant and time respectively.

Instantaneous rate of reaction can be determined graphically. Slope of the tangent at any time t gives the instantaneous rate of reaction. For a general reaction A → B in which the concentrations of A changes with time as given in the graph:

From the graph,

tan (180  –  θ) = – tan θ = – slope 

= – (OA/OB)

=> ROR (inst)

= – d[R] / dt.1  

= – (0.0 – 0.9) / (20 – 0) 

= 0.045 mol L^-1 min^–1

Similarly the instantaneous rate of reaction w.r.t. a product can be obtained from the positive slope of the curve. 

=> Instantaneous rate of reaction 

= ROR (inst) = + d[P] / (dt . ν)

 Rate of appearance, Rate of disappearance and rate of reaction:

            The rate of disappearance of a reactant is defined as the decrease in concentration of the reactant per unit time. 

ROD = Decrease in concentration of the reactant / time taken

            The rate of appearance of a product is defined as the increase in concentration of the product per unit time. 

ROA = Increase in concentration of the product / time taken          

The rate of appearance, disappearance and rate of reaction are all identical for a reaction having same stoichiometric coefficient of all the reactants and products. e.g., A + B → C + D

But for a reaction of the type 

aA + bB →  cC + dD 

where the stoichiometric coefficients are not the same, the rate of appearance, disappearance and rate of reaction are not identical.

In this case the rate of reaction 

= –1/a  (Δ[A] / Δt)  

= –1/b  (Δ[B] / Δt) 

       = 1/c  (Δ[C] / Δt)  

       = 1/d  (Δ[D] / Δt) 

Where, 

–(Δ[A] / Δt) 

= rate of disappearance of A and 

–(Δ[B] / Δt) 

= rate of disappearance of B

Δ[C] / Δt = rate of appearance of C 

and Δ[D]/ Δt = rate of appearance of D

Qulem – 1: For the given reaction, N₂ + 3H₂  —> 2NH₃, the rate of appearance of ammonia is 2 X 10^-4 M S^-1. Determine the rate of disappearance of N₂ and H₂. [Sometimes rate of disappearance of N₂ is written as rate of reaction in terms of N₂]

Solution:  The rate of appearance, disappearance and rate of reaction are related as:

–1/1  (Δ[N₂] / Δt) 

= –1/3  (Δ[H₂] / Δt)  

= 1/2  (Δ[NH₃] / Δt)   ……… relation 1

Given that Δ[NH₃] / Δt = ROA of ammonia = 2 X 10^-4 M S^-1

From relation 1,      

–1/1  (Δ[N₂] / Δt) = 1/2  (Δ[NH₃] / Δt)

=> – Δ[N₂] / Δt = ROD of N₂ 

= 1/2  (Δ[NH₃] / Δt) 

= 1/2 X 2 X 10^-4 M S^-1 

₌ 1 X 10^-4 M S^-1

Similarly,  

–1/3  (Δ[H₂] / Δt)  

= 1/2  (Δ[NH₃] / Δt)

=> – Δ[H₂] / Δt = ROD of H₂ 

= 3/2 (Δ[NH₃] / Δt) 

= (3/2) X 2 X 10^-4 M S^^-1 

=  3 X 10^-4 M S^^-1

Qulem – 2: In the reaction 2H₂O₂ —> 2H₂O + O₂, rate of formation of oxygen is 36 gram min^^-1. Determine the rate of formation of H₂O and rate of disappearance of H₂O₂.

Solution: The rate of formation of oxygen = 36 gram min^^-1. Since in a chemical reaction, reactants react according to their moles and also the products are formed accordingly w.r.t. their moles, we must convert 36 gram min^^-1 into moles.

Thus, 36 gram min^-1 of O₂ = 36/32 

= 1.125 mol min^^-1 

= Δ[O₂] / Δt = rate of appearance or formation of O₂.

The rate of appearance, disappearance and rate of reaction are related as:

–1/2  (Δ[H₂O₂] / Δt) 

= 1/2  (Δ[H₂O] / Δt)  

= 1/1  (Δ[O₂] / Δt)

Δ[H₂O] / Δt = Rate of formation (ROA) of H₂O 

= 2 (Δ[O₂] / Δt) 

= 2 X 1.125 mol min^^-1 

= 2.25 mol min^^-1

– Δ[H₂O₂] / Δt = rate of disappearance (ROD) of H₂O₂ 

=  2 (Δ[O₂] / Δt) 

= 2.25 mol min^^-1

Qulem – 3: In the reaction 2NO(g) + Cl₂ (g) —> 2NOCl (g) being carried out in a closed vessel, the partial pressure of NO is decreasing at a rate 160 torr/min. Calculate the rate of appearance of NOCl.

Solution: –1/2  ROD (NO) = 1/2  ROA (NOCl)  => ROA (NOCl) = 160 torr/min = 2.67 torr/sec

Qulem – 4: Balance the chemical reaction for acidic medium and determine the ratio of rate of change of H⁺ ion to the rate of change of MnO₄^–.

[Fe(H₂O)₂ (C₂O₄)₂]^^2– + MnO₄^^–  —> Mn^²⁺ + Fe^³⁺ + CO₂

Solution: The balanced chemical equation is:

[Fe(H₂O)₂ (C₂O₄)₂]^^2– + MnO₄^^–  + 8 H^⁺ —> Mn^²⁺ + Fe^³⁺ + 4CO₂ + 6 H₂O

For the above reaction, 

–1/1 ROD (MnO_4­^^–) = 1/8 ROD (H^⁺)

=> ROD (H^⁺) / ROD (MnO₄^^–) 

= 8/1 = 8:1

Factors affecting the rate of chemical reaction: Following factors are responsible:

1.Concentration or partial pressure of reactants:  Higher is the concentration or the partial pressure of the reactants greater is the number of molecules of the reactants. Hence greater will be the number of effective collisions between them which results in the increasing rate of reaction.

This has been mentioned also in law of mass action that the rate of chemical reaction is directly proportional to the product of concentration of reactants.

For example, a burning object burns rapidly in a gas jar full of oxygen.

2.   Temperature: We know kinetic energy increases as the temperature increases. Thus, higher is the temperature, greater will be the number of collision having sufficient kinetic energy. Hence the rate of reaction increases.

It has been found experimentally that rate of reaction increases around 2 times for each 10 degree centigrade rise in temperature. The ratio  of  specific reaction rates or  the ratio of  the rate constants  at  two  different  temperatures differing by 100c  is  called  the temperature coefficient.

Thus T.C = K (t + 100c) / K (t 0c)

            For example, copper turnings do not react with nitric acid at temperature but on heating they react vigorously.

3.   Catalyst:  A  positive  catalyst  increases  the  rate  of  reaction  by  providing  an  alternative pathway in which the activation energy is low. Hence a large number of molecules cross this energy barrier and give more products.

       For example, hydrogenation of ethane gives good yield of product in presence Ni catalysts.

       C₂H₄ + H₂ ------> C₂H₆

       But a negative catalyst decreases the rate of the reaction as it increases the activation energy.

       For example, the decomposition of H₂O₂ becomes slower in presence of phosphoric acid.

4.   Surface  area  of  reactants:  

When surface  area  of  the  reactants  increase, large  number  of molecules   are   exposed   to   the   reaction   conditions.   Thus   processes   like   combustion, adsorption etc. becomes easier. Thus higher is the surface area higher is the rate of reaction.

For example, Zn dust reacts faster with HCl than Zn granules.

5.   Nature of reactants: Reactant molecules with strong chemical bonds require higher energy to break these bonds hence the corresponding reaction becomes slower. On the other hand reactant molecules with weak bonds decompose easily, and react faster.

            A reaction which involves less bond rearrangement will be faster than the one in which there are more bond rearrangement.

            Reaction also becomes faster when they are in gaseous state than when they are solid state.

6.   Radiation: The light radiation of specific wavelength is energetic enough to break the chemical bonds of certain chemicals. Reactions carried out using photons (light radiation) are called photochemical reactions.  Thus radiations also increase the rate of reactions.

            For example, H2 and Cl2 react slowly in dark but rapidly in presence of light.

Rate law or rate equation and rate constant:  The actual relationship between the rate of reaction, concentration  of  the  reactants  and  the  exponents  on  the  reactant  concentrations  determined through experiments is called the rate law or rate equation.

The proportionality constant of the rate equation is called the rate constant. As long as the temperature remains constant and no catalyst is used, the rate constant remains constant. When temperature increases the rate of reaction also increases and accordingly the rate constant also increases. Thus rate constant is directly proportional to the rate of reaction.

  Consider the reaction, 

2NO₂ + F₂  —> 2NO₂F, 

its rate of reaction is determined experimentally and is expressed as, r = K [NO₂] [F₂]. It should be kept in mind that, the stoichiometric coefficient has nothing to do with the rate of reaction. The exponent or power of the concentration terms (1 in both [NO₂] and [F₂]) are written according to the experimental data. Here K is called the rate constant.

Order of a reaction: 

The sum total of the exponents (or powers) to which the concentration terms are raised in the rate law expression or rate equation is called the order of the reaction. The power of the concentration term of a particular reactant in the rate law is called the order of the reaction with respect to that reactant or partial order of a reactant.

If for the reaction 

mA + nB —> products, the rate of the reaction is given by r = k [A]m [B]n, then the overall order of  reaction = m + n. Also the partial order of the reaction with respect to the reactant A is m and w.r.t. B is n. 

It should be noted that, for simple or elementary reaction, which takes place in a single step without the formation of any intermediate, the partial order of the reaction is equal to the stoichiometric coefficient (smallest integers).

The reactions which take place in more than one step and in which at least one intermediate is formed are called the complex reactions. For these reactions, the partial order may or may not be equal to the stoichiometric coefficient.

 Order of reactions can be of the following types:

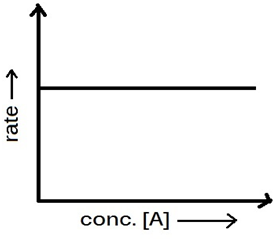
(1)  Zero order reaction: The reaction in which the rate of reaction is independent upon the initial concentration of reaction is called zero order reaction. In general, reactions taking place on the surface of the catalysts or in presence of sunlight are zero order.

Example:1   

2NH3  — > N2  + 3H2    

(this reaction takes place over Pt catalyst) Rate = K [NH3]^0 

2. Reaction of hydrogen and chlorine in presence of sunlight to give hydrogen chloride is also a zero order reaction. Rate = K [H₂]⁰ [Cl₂]⁰

(2)  First order reaction: In this type of reaction, the rate of reaction depends only upon the concentration of one reacting species, raised to the power unity.

Example:1   

PCl₅    —>  PCl₃ + Cl₂  ;  

rate = — d [PCl₅] / dt 

=> r = K [PCl₅]^¹

(3)  Pseudo first order reaction: The reaction in which there are more than one reactants but the rate of reaction depends only on the conc. of one reactant species, raised to the power unity is called pseudo first order reaction. All other reactants being present in excess do not affect the rate of reaction.

Example: Hydrolysis of ethyl acetate with water.

CH₃COOC₂H₅ + H₂O —> CH₃COOH + C₂H₅OH

Rate = K [CH₃COOC₂H₅]^1    

=> Order = 1         

 (4)  Second order reaction: 

The reaction in which the rate of reaction depends upon the molar concentrations of two reacting species each raised to the power equal to unity or the reaction in which the rate of the reaction depends on one reacting species raised to the power equal to two is called a second order reaction.

EX : Hydrolysis of ethyl acetate with NaOH.

CH3COOC2H5 + NaOH —> CH3COONa + C2H5OH

R = K [CH3COOC2H5]^1 [NaOH]^1      => Order of reaction = 2

(5)  Fractional order reaction: The reaction in which the rate of reaction depends upon the molar concentration of reacting species having fractional power.

Example:

CH3CHO (l) —> CH4 (g) + CO (g), 

r = K [CH3CHO]^3/2   , 

order = 3/2

Characteristics of order of reaction:

i.It depends upon the conditions of the reaction

ii.It may be zero, whole number or a fraction.

iii.It can be determined only experimentally

iv.The overall order of any reaction is always positive though partial order may be negative.

v.In case a reaction is complex and proceeds in several steps, the order of the reaction is determined from the slowest step. (Slowest step is the rate determining step)

 Qulem – 5: If 2A + 4B —> products is an elementary reaction, then what will be its order?

Solution: In an elementary reaction smallest possible integer of stoichiometric coefficients are taken.

2A + 4B —> products becomes A + 2B —> products

Thus r = K [A] [B]^² and order = 1 + 2 = 3

Qulem – 6: The reaction 2NO (g) + Cl₂ (g) —> 2NOCl (g) has an observed rate law, r = K [NO]^² [Cl₂]. Predict whether the reaction is simple or complex.

Solution: Since the partial orders of the reaction are similar to the stoichiometric coefficients, the reaction may be an elementary or may be a complex reaction.

Rate constant or specific reaction rate:

Consider a reaction A + B —> Product

Rate of reaction, 

r = dx /dt =  K [A] [B] Where k is the proportionality constant or the rate constant

When [A] = 1 mole/ltr and 

[B ] = 1 mol/ltr 

then rate = K = specific reaction rate

The rate constant (or specific reaction rate) of reaction at a given temperature is defined as the rate of reaction when the molar conc. of each the reacting species is unity.

Units of rate constant:

Let A —> product (a first order reaction)

r = K [A]  or  K = r / [A] 

= Mole Ltr^-1 sec^ -1 / Mole Ltr^-1   

= sec^ -1     

=> Unit of rate const. of first order reaction = sec^-1

Let A —> product (a zero order reaction)

r = K [A] ^0   => K = r 

=> Unit of K of zero order reaction 

= Mol lit^ -1 sec^-1

In general, 

Unit of rate constant ‘K’ of nth order reaction is Mol^ (1-n) litre^(n -1) sec^ -1 

Molecularity: The number of reacting species (molecules \atoms\ions) taking part in an elementary reaction to bring about the product is called the molecularity. This is a theoretical concept defined only for elementary reactions.

If the decomposition of a single species brings about the product then it is a unimolecular reaction.  i.e., the number of reacting species = 1,

For Ex: NH4NO2 —> N2 + 2H2O,    

or     N2O5   —> N2O4  + ½ O2,         The molecularity = M = 1

For any reaction of the type: 

A+B —> product, 

Molecularity = M =2 (called bimolecular eaction)

For Ex: CH3COOC2H5 + NaOH —> CH3COONa + C2H5 OH

For any reaction of the type: 

A+B+C —> product, or 2A + B —> product, M = 3 (called trimolecular reaction)

For Ex: 2SO2 + O2 —> 2SO3

Characteristics of molecularity:

1.   Molecularity is determined for a single step reaction or a single step of a complex reaction.

2.   It is always a whole number and can’t be fraction, zero or negative.

3.   The value of molecularity can’t exceed 3. 

In case a reaction contains more than 3 reactants, it  can’t  takes  place  in  a  single  step  and  proceed  through  two  or  more  consecutive  steps. These types of reactions are called complex reactions.

Consider a reaction: 

4HBr + O2  —> 2H2O + Br2  which occurs stepwise as follows:

HBr + O2  —> HOOBr (slow)

HOOBr + HBr —> 2HOBr

HOBr + HBr —> H2O + Br2

Since the slow step contains two molecules, its molecularity is 2. Here molecularity of the overall reaction has no significance.

Qulem – 7: You have an elementary reaction of the type 

A + 2B —> Product. What happens to the rate of reaction if volume of the vessel is reduced to one third of its initial volume?

Solution: For an elementary reaction the partial orders are equal to the stoichiometric coefficients.

Thus for A + 2B —> Product, the rate, Rate1 = k [A] [B]^²

Concentration = mole/Volume. Thus when volume is reduced to one third the concentration becomes three times.

The final Rate2 = k [3A] [3B]^² 

= 27 k [A] [B]^²

Now Rate2 / Rate1 = 27. Thus the final rate becomes 27 times faster than the initial rate.

Qulem – 8: Given a reaction 

2NO (g) + Cl₂ (g) —> 2NOCl (g.  The rate of reaction is doubled when concentration of Cl₂ is doubled and it becomes eight times when concentration of both NO and Cl₂ are doubled. Predict the order of reaction.

 Solution: Let partial order of the reaction with respect to NO is X and w.r.t. Cl₂ is Y.

Rate = r = K [NO]^^X [Cl₂]^^Y

Now according the question, 2r = K [NO]^^X [2Cl₂]^^Y and  8r = K [2NO]^^X [2Cl₂]^^Y

Solving the above equation we get X = 2 and Y = 1, Hence the overall order of the reaction is = 2+1 = 3

Integrated rate equation:

Till now we have expressed the concentration dependence of rate as differential rate equation. When we integrate the differential equation, we get a direct relationship between rate of reaction, molar concentration, rate constant and time. Such equations are called integrated rate equation.

Derivation of the rate constant of a zero order reaction or Integrated rate equation for zero order reaction:

Consider a zero order reaction,

Rate of reaction = — d [a —  x] / dt 

=  dx / dt = K0 [A]^ 0 

= K (a — x) ^0 = K 

Where K = rate constant for zero order reaction. Thus dx = K₀ dt

Integrating both sides,

=> x = Kt         => K = x/t  …….. eq 1.    This is called the integrated rate equation for the zero order reaction where x is the amount of reactant consumed or the amount of product formed in time t.

If [A]0  = initial concentration of A (at t =0) and [A]t  = concentration of A at any time t, then eq. 1 becomes 

K = {[A]0 — [A]t} / t  

=> [A]t = — Kt + [A]0   …….. eq. 2 

 or     [A]₀ — [A]t = Kt

Half life period (t_{1/2 or} t_50%): 

The time in which half of the reaction is complete is called the half life period. From eq. 1, t = x / K, 

when t = t_1/2  then x = a/2  

=> t_1/2  = a / 2K, which shows that the half life period of a zero order reaction is directly proportional to the initial concentration.

The following graphs relate various parameters of zero order reaction:

  

 Characteristics of Zero order reaction:

1. Zero order reactions proceed with constant rate.

2.Half life of these reactions is directly proportional to the initial concentrations and inversely proportional to the rate constant.

3.Rate of reaction does not change with change in concentration of the reactant.

4.The unit of rate constant is same as that of the rate of reaction, mol L^-¹ S^-¹

Derivation of the rate constant of a first order reaction or integrated rate equation for first order reaction:

Consider a first order reaction,

Rate of reaction =  dx / dt = K0 [A]^ 1 

= K (a — x)1 = K (a — x)

Where K = rate constant for first order reaction.

Thus dx / (a — x) = K dt

Integrating in both sides,

∫ dx  / (a —  x) = ∫ K dt

=>  —  ln  (a  –  x)  =  Kt  +  C  …….  Eq.1, 

where  C  is  the  integration  constant,  whose  value  can  be determined as follows,

When t = 0, x = amount of reactant consumed = 0

=>  —ln a = C

Putting the value of C in eq. 1, we get

— ln (a – x) = Kt — ln a

=> ln a – ln (a — x) = Kt            

=> ln (a — x) = – Kt + ln a …….. eq. 2

=> ln {a / (a — x)} = Kt                         

 => a / (a — x) = Exp (Kt)  ……. Eq. 3

=> 2.303 log {a / (a — x)} = Kt  

=> (a — x) = a Exp (— Kt)  ……. Eq. 3a

=> K = (2.303 / t) log {a / (a — x)} ……. eq. 4. 

This is called the integrated rate equation for the first order reaction where x is the amount of reactant consumed or the amount of product formed in time t.

Half life period (t_1/2): 

The time in which half of the reaction is complete is called the half life period. When t = t_1/2  then x = a/2, putting the value of x in eq. 3, we get,

K = (2.303 / t_1/2) log {a / (a/2)}             => K = (2.303 / t_1/2) log 2         

=>  t_1/2   =  0.693  /  K  ……..  eq.  4, which  shows  that  the  half  life  period  of  a  first  order  reaction,  does  not  depend  on  the  initial concentration. The following graphs relate various parameters of first order reaction:

  

Kinetics of first order reaction in terms of partial pressure:

Consider a general gaseous first order reaction of the type 

A(g) —> B(g) + C (g)

Let Pi be the initial pressure in the vessel due to A only and the total pressure at any time t be Pt and the partial pressure of A, B and C be P_A, P_B and Pc.

Consider that the decrease in partial pressure of A in time t is X atm.

 Total pressure at time t, 

= Pt = pi – X + X + X 

= Pi + X                         

=> X = pt – pi

And P_A = pi – X = pi – (Pt – pi) 

= 2pi – Pt

Putting these data into first order kinetics 

K = (2.303 / t) log {a / (a — x)}, we get,

K = (2.303 / t) log (pi / P_A) 

= (2.303 / t) log {(pi / (2pi – Pt)}

Characteristics of First order reaction:

1.Rate is directly proportional to the unit power of the concentration of reactant.

2.Half lives of these reactions are independent of the initial concentrations.

3.The time required for the 75% completion of the reaction is twice of its half life period.

4. The time required for the 99.9% completion of the reaction is ten times of its half life period.

5.The unit of rate constant is S^^—1

Degree of completion (α) of reaction:

The part of the reaction which is complete in time t is called the Degree of completion (α) of reaction.

α =  = X/A₀        => X = A₀α

For any reaction, 

[A]t = amount of reactant at any time t =  [A]₀ — X 

= [A]₀ — [A]₀ α 

= [A]₀ (1 — α)

The term t_α represents the time required for the completion of α part of the reaction.

For Example: The time required for the 75% completion of the reaction is represented as t_75% or t_3/4.

Qulem – 9: What is the relationship between the rate constant of a zero order reaction and the time required for the 75% completion of the reaction?

Solution: For zero order reaction, [A]₀ — [A]t = Kt    ……. eq 1

α = 75% = 3/4  

=> [A]t = [A]₀ (1 — α) 

= [A]₀ (1 — 3/4) = [A]₀/4  (Putting this value in equation 1)

=> [A]₀ — [A]₀/4  = K t_75%          

=> 3/4 [A]₀ = K t_75%     

=>  t_75% = 3[A]₀ /4K

Similarly, t_100% = [A]₀ /K = 2 t_50%

Note: When the order (n) of reaction is 0 ≤ n < 1, it proceeds to completion. All other reaction never proceed to completion.

Qulem – 10: Certain reaction A —> B follows zero order kinetics. The concentration of A drops from 3M to 1.5M in 1 hour. How much time should it take to reach at 0.25M from 0.5M?

Solution: Since concentration of A drops from 3M to 1.5M in 1 hour, its half life = t_50%  = 1 hour.

=> k = [A]₀ /2 t_50%          

=> K = 3/2 = 1.5 M/hour

Since, [A]₀ — [A]t = Kt               

=> 0.5 – 0.25 = 1.5 X t    

=> t = 0.167 hour

 Qulem – 11: Show that the time required for the completion of three fourth of a first order reaction is twice of that needed for the completion of half of the reaction.

Solution: Take ratio for t_3/4 and t_1/2for first order reaction and proceed.

Qulem – 12: A substance S having half life of 100 minutes reacts following first order kinetics. Calculate the fraction of initial concentration of S, which will be reacted in 300 minutes.

Solution: We need 

{a — (a  — x)} / a = ?

For first order reaction,  

(a — x) / a  =  Exp (— Kt)

Half life =  100 minutes  

=> K = (ln 2)/100

Thus (a — x) / a = Exp (— Kt)   

=> (a — x) / a 

= Exp [{(—ln 2)/100} 300] 

= 2^^—3 

= 1/8

Hence fraction of initial concentration reacted = 1 – 1/8 = 7/8

Or

For first order reaction, the amount of substance left after n half lives, 

N = N₀ (1/2)^ⁿ

Since in the above reaction half life = 100 minutes          => 300 minutes = 3 half lives      => n = 3

=> N = N₀ (1/2)^³ = N₀ / 8

Thus he required answer, (N₀ – N) / N₀ = 1 – 1/8 = 7/8

Qulem – 13: The decomposition of N₂O₅ (g) into NO₂ (g) and O₂ (g) follows first order kinetics, in which the rate constant is 7.48 X 10^-3 S^^-1. If initially only N₂O₅ was present at 0.1 atm, determine the time needed to raise the pressure to 0.145 atm.

Solution:

The total pressure = Pt = P0 – 2P + 4P + P = P0 + 3P             

=> 2P = (2/3) (Pt – P0)

The partial pressure of N₂O₅ = P0 – 2P = P0 – (2/3) (Pt – P0)

We are to raise the total pressure to 0.145 atm. Thus when Pt = 0.145 atm,

The partial pressure of N₂O₅ = P0 – (2/3) (Pt – P0) = 0.07 atm

Applying kinetics equation w.r.t. pressure, t = (2.303 / k) log (P0 / P_A)

=> t = (2.303 / 7.48 X 10^-3 S^-1) log (0.1 / 0.07) = 47.7 sec

Some important first order reaction and their experimental measurement:

1. Decomposition of Hydrogen peroxide in water: H₂O₂ decomposes in water in presence of finely divided Platinum following first order kinetics.

H₂O₂ —> H₂O + ½ O₂

As the reaction proceeds the concentration of H₂O₂ decreases and that of oxygen increases. Accordingly the progress of the reaction can be measured by titrating equal volume of reaction mixture (withdrawn at regular intervals) against a standard KMnO₄ solution.

Volume of KMnO₄ consumed at the beginning of reaction can be considered as the initial concentration of the H₂O₂ and accordingly the volume of KMnO₄ at any time t can be considered to be the remaining unused H₂O₂.

Thus V₀ = The volume of KMnO₄ used at the beginning ≡ Initial concentration of H₂O₂ ≡ a

And V_t = The volume of KMnO₄ used at different time interval ≡ Amount of unused H₂O₂ ≡ a — X

Qulem – 14: Following is a set of data for the decomposition of H₂O₂. Show that the reaction is of first order. 

Time (s)	0	600	1200
KMnO4 (ml)	22.8	13.8	8.3

 Solution: For first order reaction, K = (2.303 / t) log {a / (a — x)}

Here V₀ = a = 22.8 ml and V_t = a — x  = at 600 sec = 13.8

and at 1200 sec = 8.3 ml

At 600 sec

K = (2.303 / 600) log (22.8 / 13.8)

For 1200 sec

K = (2.303 / 1200) log (22.8 / 8.3)

Find the value of K in both of these two cases. You will find K in both of the cases equal or nearly equal which will prove it to be the first order.

2. Hydrolysis of methyl or ethyl acetate: In this case, the progress of the reaction is measured in terms of formation of product. The formation of acetic acid is measured by titrating against standard NaOH solution.

CH3COOR + H2O —> CH3COOH + ROH

In such case, initial concentration of the ester ≡ concentration of acetic acid measured at end of the reaction — concentration of acetic acid measured initially 

≡ V_∞ — V₀  ≡ a

And V_∞ — V_t ≡ a — X

3. Decomposition of N₂O₅ in CCl₄:

In this case, the volume of oxygen is measured to follow the progress of the reaction.

N₂O₅ —> N₂O₅ + ½ O₂

N₂O₄ —> 2NO₂

In this case, V_∞ ≡ Volume of oxygen collected at the end of reaction (infinite time)

Initial amount of N₂O₅ ≡ a ≡ V_∞ and

V_t ≡ Volume of oxygen collected at time t ≡ X and thus V_∞ — V_t ≡ a — X

Now we can apply the formula: 

K = (2.303 / t) log { V_∞/ (V_∞ — V_t)}

4. Inversion of cane Sugar: C₁₂H₂₂O₁₁ + H₂O —> C₆H₁₂O₆ + C₆H₁₂O₆ . In such case, the progress of the reaction is studied by measuring the angle of rotation of the reaction mixture. Sucrose is dextro-rotatory where as the mixture product is laevo-rotatory. This conversion from d- to l- (positive to negative angle of rotation) is called inversion of sugar.

r₀ — r_∞ ≡ a;     r₀ — r_t ≡ X;      

r_t — r_∞ ≡ a — X

Now we can apply the formula: 

K = (2.303 / t) log {(r₀ — r_∞) / (r_t — r_∞)}

Qulem – 15: The optical rotation values in the inversion of sucrose using 0.55N HCl at 27⁰C is given below. Find out the order of the reaction. 

Time (min)	0	10	20	40	∞
Rotation (Degrees)	+32.4	+28.8	+25.5	+19.6	—11.1

5. Radioactive Decay: The radioactive disintegrations follow first order kinetics.

Qulem – 16: The half life of C^¹⁴ isotope is 5730 years. An wooden vessel found from certain archeological activity has only 80% of the C^¹⁴ found in a freshly cut wood. Estimate the age of the sample.

Solution: Take a = 100 and a — X = 80. Calculate K from half life and put the formula to get answer

nth order reaction: For any reaction of order n, we can derive the integrated rate equation:

(n — 1) K.t 

= {1/A_t^^(n—1)} — {1/A₀^^(n—1)} (Except n = 1)

Putting A_t = A₀ (1 — α) we have,

t_α  = {1/(A_0­^^(n—1)} [(1/k(n—1){(1/(1 — α) ^^(n—1)) —1}]

Accordingly, for a second order reaction (n=2), we have,

Kt = (1/A_t) — (1/A₀) and t_1/2 = 1/ A₀K

Collision theory of reaction rate:  According to collision theory,

Reactants are made up of molecules and are always in a state of random motion.

They go on colliding with one another.

 The collision frequency (Z) is the number of intermolecular collisions per unit volume per second at a   given temperature.

Any collision which is effective brings about the chemical reaction. A collision becomes effective when it satisfies the following conditions:

i.The molecules colliding should possess energy equal to or greater than a certain minimum value of   energy known as threshold energy. This energy is related to the activation energy.

     ii .They should have proper orientation.

Collision theory can be discussed for two cases:

1.       Lindemann’s Theory:

Lindemann proved how some reaction seems to be bimolecular but follow first order kinetics.

Consider a reaction A ---------> Products

Lindemann proposed the following mechanism:

A+A ---------> A* + A (Activation)  ------- i

A +A* ----------> A + A (Deactivation) ------- ii

A* -----------> Products (Reaction)  --------- iii

Lindemann explained that there exists a time lag between the activation and reaction.

In case the time lag is long, then step iii is slow and the reaction follows first order kinetics but if the time lag is short, then step ii is slow and the reaction will follow second order kinetics.

Lindemann also explained how pressure affects kinetics of reaction.

2.                  For an elementary bimolecular reaction:

A + B —> product

If Z AB  is the collision frequency and

If ‘f’ is the fraction of collisions between molecules which possess energy equal to or greater than a certain minimum value of energy known as activation energy,

Then the rate of reaction, r = dx/dt  ∝  ZAB x f ……….eq. 1

Further according to kinetic energy of gases,

The fraction of molecules which possess energy equal to or greater than a certain minimum value of energy known as activation energy,  ‘Ea’ at a temperature T is given by Boltzmann factor (f).

f = e^—Ea/RT

Thus substituting for ‘f’ in eqn (1) we get

r = rate  ∝  ZAB   x e^—Ea/RT

As rate of reaction is directly related to a rate constant ‘K’ thus we can write

K ∝ ZAB x e^—Ea/RT   ---------  eq. 2

  To account for the orientation factor (for making the collision effective) another factor (P) called orientation   factor or probability factor should be introduced.

Hence, the eq (2) becomes K = P. ZAB x e^— Ea/RT

 

Limitations of collision theory:

1.   For some reactions, the calculated and experimental value of K very widely

2.   It fails to explain rate of reversible reaction

3.   It is not possible to predict the correct orientation before the reaction collide effective

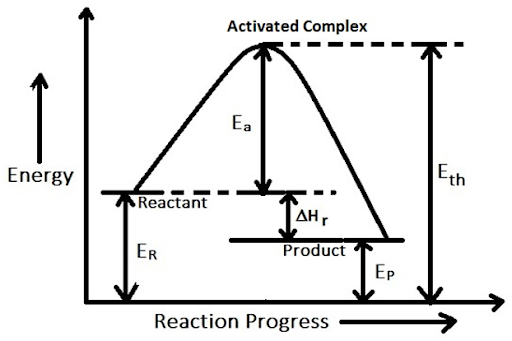
Concept of activation energy and activated complex:

The reactants in a chemical reaction must acquire the threshold energy 

to bring about the product. The extra energy supplied to the reactant to attain the threshold energy and to undergo the chemical reaction is called the activation energy.

Activation (Ea) = Threshold (Eth) — Average kinetic energy of reactant (E R)

To illustrate the concept of activation energy, we can take the example of the reaction between methane and oxygen. They don’t react at ordinary temperature even though they collide with each other because they posses energy less than threshold energy.  But when a lighted match stick is brought near the reaction mixture they gain additional energy to reach threshold energy and begin to react. The heat produced help in continuing the reaction of other molecules.

The  value  of  activation  energy  decides  the  fraction  of  total  number  of  collisions  which  is effective. Clearly if the value of activation energy is low, large number of molecule will possess this energy. Hence the number of effective collisions will be more. So fast reactions have low activation energy and slow reaction have high activation energy.

            For Example, even though concentration and temperature remains constant, rate of reaction varies from reaction to reaction. For example,

NO (g) + ½ O₂ (g) ---------> NO₂ (g)    (Fast)

CO (g) + ½ O₂ (g) ---------> CO₂ (g)    (Slow)

Since the bond in CO is stronger than NO, the activation energy of CO is greater than NO. Hence the oxidation of CO is slower than that of NO.

Exothermic and endothermic reaction:

Exothermic reaction:  In exothermic reaction, energy of product is less than that of reactant.

Thus the enthalpy of the reaction, 

ΔH r   = ΣHP   —  ΣHR   =  — ve . That means energy is lost.

Endothermic reaction:  In endothermic reaction, energy of product is greater than that of reactant.

Thus the enthalpy of the reaction, 

ΔH r   = ΣHP   —  ΣHR   =  + ve . That means energy is gained.

Activated complex:  An  activated  complex  is  an  intermediate  state  that  is  formed  during  the conversion  of reactants into products. It is the structure that results in the maximum energy point in the reaction path. An activated complex may give rise to product or may be deactivated to the reactant.

For the reaction between H₂ and I₂ we have, H₂ + I₂ —> 2HI

Activation energy in reversible reaction:

In reversible reaction,

Activation energy (Ea) for forward reaction = Ea (forward) = Eth — ER 

Activation energy (Ea) for backward reaction = Ea (backward) = Eth — Ep

If Ea (forward) > Ea (backward), then reaction is endothermic.

If Ea (forward) < Ea (backward), then reaction is exothermic.

Effect of temperature on the rate of reaction:

It  has  been  already  been  explained,  for  most  of  the  reaction,  the  rate  of  reaction  comes nearly  double  or  even  more  for  10  0c  rise  in  temperature.  This is explained in equation as temperature co efficient = K (t+10) / K (t0c) = 2 to 3

Explanation:

This can be explained considering the increase in effective collision. The following graph makes it clear,

We  know  from  kinetic  theory,  kinetic  energy  is  directly  proportional  to  the  temperature. From the above graph of molecular velocity, the point ‘a’ represents the minimum kinetic energy that should be possessed by molecule for effective collision. The area ‘abcd’ represents the total number of molecules having energy greater than the threshold value at temperature t0c.

When the temperature is increased to (t + 10) 0c, the curve shifts to the area ‘abef’. Thus the fraction of molecules having kinetic energy greater than threshold value is now almost double than the area abcd. Hence the increase in rate of reaction with increase in temperature is mainly due to the increase in the number of effective collision.

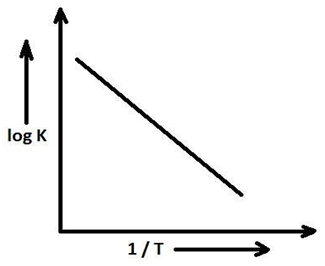
Arrhenius equation:  

A  quantitative  approach  which  relates  the  rate  of  reaction  (rate  constant), temperature and activation energy is given by Arrhenius known as Arrhenius equation.

The Arrhenius equation is given by,

K = A e ^— Ea/ RT  ……….eq. 1  Where K = Rate constant

A = frequency factor

Ea  = Activation energy

R = universal gas constant

T = temperature in Kelvin

Taking logarithm in both sides,

ln K = ln A e ^— Ea/ RT

=> ln K = ln A + ln e ^— Ea/ RT

=> ln K = ln A — Ea/ RT

=>  2.303 log K = 2.303 log A – Ea / RT

=> log K =  log A – Ea  / 2.303 RT ----------- eq. 2

When a graph is plotted for log K vs ‘1 / T’ we get a straight line where the intercept is ‘log A‘ and the slope is  — Ea  / 2.303R. 

If K1 and K2 are the two rate constants at two different temperatures T1  and T2  respectively, then,

log K1  = log A – Ea  / 2.303RT1   -------- eq.3

& log K2  = log A – Ea  / 2.303RT2  -------- eq. 4

Subtracting eq (3) FROM (4)

log K2  – log k1 = (Ea / 2.303RT1)  – (Ea  / 2.303RT2)

log (K2 /K1) = Ea  / 2.303R (1/T1  – 1/T2)

log (K2 /K1) = Ea  / 2.303R {(T2 – T1) / T1 T2}   --------- eq. 5

The  equation  (5)  is  the  logarithmic  form  of  Arrhenius  equation  which  gives  the  variation  of  rate constant with temperature. Knowing all other values, activation energy can be calculated.

Qulem – 17: The rate constants of a reaction at 50⁰c and 100⁰c are found to be 1.5 X 10⁷ s^-1 and 4.5 X 10⁷ s^-1. Calculate the activation energy.

Solution: Put R = 8.314 j k^-1 mol^-1 and proceed

To be continued ....

A Quality book is like a best friend. Let’s enjoy the friendship.